Ciro Santilli's legs sometimes itch when he runs Updated 2025-07-16
Ciro Santilli has an undiagnozed condition where his upper legs and lower torso often start to itch when he runs, to the point of being extremely annoying and removing all pleasure form the activity.
If some doctor knows why this could be, please tell him!!!
The problem is a bit hard to reproduce however, and Ciro hasn't been able to determine which exact condition triggers it: temperature, nutrition, something else?
Ciro believes that this is not chiefly due to transpiration, but rather to the impact motion that running does on the muscles, as he has felt something similar on his arms some times while cycling in very rough terrain, which made his arms shake in a similar fashion. or for example if he has a water bottle on a tightly tied backpack that rubs his back, then the back itches at that point.
Also, running on a threadmill is not a problem at all. Ciro believes that this is because the threadmill is better amortized, and therefore does not cause the same mechanical stress required to create the itching as running on pavement.
Interestingly, Ciro didn't feel that at all when he played soccer enthusiastically as a child, and he was one of the fastest runners of the group for sure at that time. So he's not sure if it started when he got older, or if it is just because the difference in workloads between soccer and running.
youtu.be/PNgYW5N95z8?t=945 "My back itches" after riding on a bumpy makeshift motorcycle.
Hash function Updated 2025-07-16
Applications:
- hash map which is a O(1) amortized implementation of a map
- creating unbreakable chains of data, e.g. for Git commits or Bitcoin.
- storing passwords on a server in a way that if the password database is stolen, attackers can't reuse them on other websites where the user used the same password: security.blogoverflow.com/2013/09/about-secure-password-hashing/
Hazard (computer architecture) Updated 2025-07-16
Ciro Santilli's naughty projects Updated 2025-07-16
If Ciro Santilli weren't a natural born activist, he chould have made an excellent intelligence analyst! See also: Section "Being naughty and creative are correlated".
- Stack Overflow Vote Fraud Script
- GitHub makes Ciro feel especially naughty:
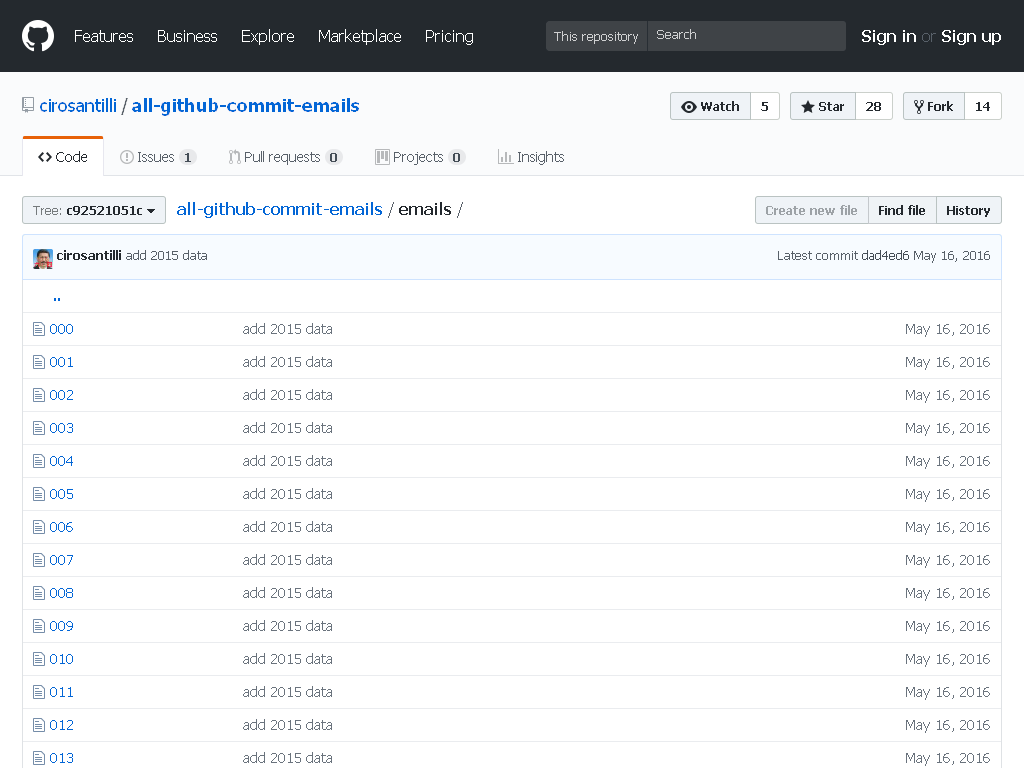
- All GitHub Commit Emails: he extracted (almost) all Git commit emails from GitHub with Google BigQuery
Figure 1. All GitHub Commit Emails repo before takedown. Screenshot from archive.is. - A repository with 1 million commits: likely the live repo with the most commits as of 2017
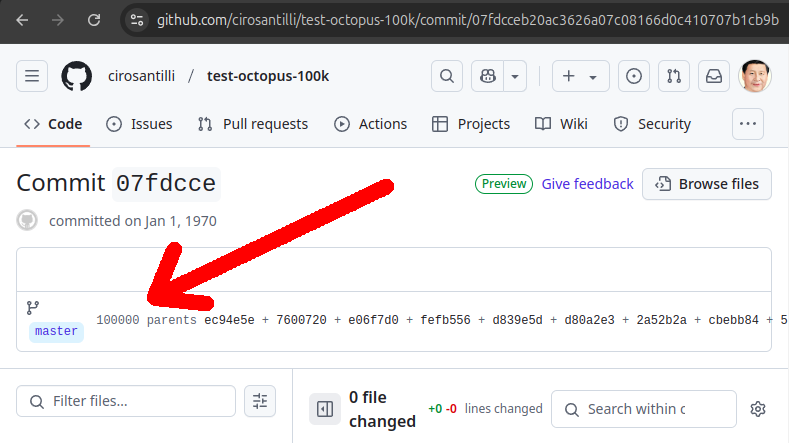
- An 100 year GitHub streak, likely longest ever when that existed. It was consuming too much server resources however, which led to GitHub admins manually turning off his contribution history.
Figure 3. Screenshot of a commit with 100k parents on GitHub. URL: github.com/cirosantilli/test-octopus-100k/commit/07fdcceb20ac3626a07c08166d0c410707b1cb9b- 500 on adoc infinite header xref recursion: that was fun while it lasted
Ciro Santilli's open source contributions Updated 2025-07-16
Ciro Santilli's open source contributions Merged by Ciro Updated 2025-07-16
Patches which were merged by Ciro himself on repositories which eh feels have large public visibility, e.g. those to which he has been given push permission.
Repositories to which Ciro gained push permission because of his contributions:
Ciro Santilli's open source contributions Security Updated 2025-07-16
| Date | Project | Size | Description |
|---|---|---|---|
| 2016-05 | All GitHub Commit Emails | 1 | Password disclosure grep password on email data. Gmail password worked and user confirmed. |
Heat equation Updated 2025-07-16
Besides being useful in engineering, it was very important historically from a "development of mathematics point of view", e.g. it was the initial motivation for the Fourier series.
Some interesting properties:
- TODO confirm: for a fixed boundary condition that does not depend on time, the solutions always approaches one specific equilibrium function.This is in contrast notably with the wave equation, which can oscillate forever.
- TODO: for a given point, can the temperature go down and then up, or is it always monotonic with time?
- information propagates instantly to infinitely far. Again in contrast to the wave equation, where information propagates at wave speed.
Sample numerical solutions:
Heroku Updated 2025-07-16
This feels good.
One problem though is that Heroku is very opinionated, a likely like other PaaSes. So if you are trying something that is slightly off the mos common use case, you might be fucked.
Another problem with Heroku is that it is extremely difficult to debug a build that is broken on Heroku but not locally. We needed a way to be able to drop into a shell in the middle of build in case of failure. Otherwise it is impossible.
Deployment:
git push heroku HEAD:masterView stdout logs:
heroku logs --tailPostgreSQL database, it seems to be delegated to AWS. How to browse database: stackoverflow.com/questions/20410873/how-can-i-browse-my-heroku-database
heroku pg:psqlDrop and recreate database:All tables are destroyed.
heroku pg:reset --confirm <app-name>Restart app:
heroku restart Ciro Santilli's selfish desires Updated 2025-07-16
Just enough money to raise 3 kids in a rich country without having to work (so he can focus on whatever project he wants) and no more. Then maximize fame.
Fame is slightly convertible into money with generally little liquidity, but is more valuable if money becomes useless in a TEOTWAWKI.
Of course, in the end, one just does whatever seems cool and useful, and the Gods decide what proportion of fame/money/power they will get. Due to Ciro's love of open source software however, a higher fame percentage seems more likely than money.
Searching just for just "Santilli" on Google does not give any Ciro Santilli hits. The name appears to be a minor variation of the much more common "Santini". Since the name is not that common, it is possible to go over all noteworthy hits. Some relevant ones are shown at: interesting members of the Santilli family.
Searching just for just "Ciro" on Google does not give any Ciro Santilli hits, mostly some smaller brands that could be beaten, this is Ciro's main initial fame metric goal. Reaching it would require doing things known much beyond the programming community however, as Ciro has done until of 2019. ciro.com is from an electromechanics consultancy as of 2019, so it's not bad, let them be.
At the next useless gamified level, an honorary OBE and more ambitiously ForMemRS from the Royal Society post nominal letters would be nice.
The ultimate dream however would be to beat Cyrus the Great himself on Google searches ("Ciro" == "Cyrus" in Portuguese), maybe becoming "Cyrus the Greater"? That one will be a bit harder though. Maybe if Falung Gong becomes the dominant religion in 2000 years like Christianism did, catapulting the Judaism benefactor Cyrus into greater fame, then there is some hope for Ciro as well.
Ciro Santilli's sport practice Updated 2025-07-16
As a Brazilian, Ciro Santilli used to really love playing soccer (but not watching it), until he hurt his knee.
Playing soccer just feels amazing, because you are constantly running around, but with a more specific goal in mind: to get that ball into that goal!
Playing soccer was specially amazing in the flat wet sand beach of Santos. weekend, the sea, feet touching the sand, the sun going down, and your school mates next to you. Nirvana.
It is also true that under those conditions, the skin of your feet will get ripped off due to running on the slightly wet and flat sand no matter how thick it has become. But it is worth it.
Teams would often be slit between "the team with shirts vs the team without shirts", who would just take off their shirts. The two best players would take turns picking players into their teams, the first one to pick would be decided by odds and evens (par ou ímpar).
A pair of Havaianas, or Havaianas rip-offs, stuck into the sand, or even just some school bags, would do as a goal posts. More organized people, especially adults, would have their own water pipe goal with a proper net and all. But doing so would spoil the fun of endless discussions if a non flat ball had gone in or not into an imaginary rectangle.
That's how soccer was meant to be played.
Ciro hates water, so swimming is out of the question. What could be more boring than going back and forth on a fixed location a million times to gain some milliseconds?
Running would have been a consideration, but Ciro Santilli's legs sometimes itch when he runs.
This is until he ended up living in a place with decent roads for cycling in the late 2010's, which led to Ciro Santilli's cycling.
Ciro Santilli's undergrad studies at the University of São Paulo Updated 2025-07-16
Ciro's official diploma from the University of São Paulo read "Automation and Control Engineer at the Polytechnic School of the University of São Paulo".
The University of São Paulo had been elected the best South American university in the Times Ranking 2013 (archive) in all subjects.
Ciro finished the course with honors of "The Best Student in Automation and Control of the year 2013".
Ciro didn´t learn basically any control engineering however unfortunately. He did only the 3 base years of the electrical engineering course, and the rest got lost on stupid politics of having to go back to do 6 months from France to validate his Brazilian degree, see also: Section "Don't force international exchange students to come back early".
Ciro's call hierarchy notation Updated 2025-07-16
This is a simple hierarchical plaintext notation Ciro Santilli created to explain programs to himself.
It is usuall created by doing searches in an IDE, and then manually selecting the information of interest.
It attempts to capture intuitive information not only of the call graph itself, including callbacks, but of when things get called or not, by the addition of some context code.
For example, consider the following pseudocode:Supose that we are interested in determining what calls
f1() {
}
f2(i) {
if (i > 5) {
f1()
}
}
f3() {
f1()
f2_2()
}
f2_2() {
for (i = 0; i < 10; i++) {
f2(i)
}
}
main() {
f2_2()
f3()
}f1.Then a reasonable call hierarchy for
f1 would be:f2(i)
if (i > 5) {
f1()
f2_2()
for (i = 0; i < 10; i++) {
f2(i)
main
f3
f3()
main()Some general principles:
City in Japan Updated 2025-07-16
City of London Updated 2025-07-16
The City of London is an obscene thing. Its existence goes against the will of the greater part of society. All it takes is one glance to see how it is but a bunch of corruption. See e.g.: The Spiders' Web: Britain's Second Empire.
Classical limit Updated 2025-07-16
The idea tha taking the limit of the non-classical theories for certain parameters (relativity and quantum mechanics) should lead to the classical theory.
It appears that classical limit is only very strict for relativity. For quantum mechanics it is much more hand-wavy thing. See also: Subtle is the Lord by Abraham Pais (1982) page 55.
Classical mechanics Updated 2025-07-16
Classification (mathematics) Updated 2025-07-16
Examples:
- classification of finite simple groups
- classification of regular polytopes
- classification of closed surfaces, and more generalized generalized Poincaré conjectures
- classification of associative real division algebras
- classification of finite fields
- classification of simple Lie groups
- classification of the wallpaper groups and the space groups
Classification of 4-transitive groups Updated 2025-07-16
en.wikipedia.org/w/index.php?title=Mathieu_group&oldid=1034060469#Multiply_transitive_groups is a nice characterization of 4 of the Mathieu groups.
Classification of 5-transitive groups Updated 2025-07-16
Apparently only Mathieu group and Mathieu group .
www.maths.qmul.ac.uk/~pjc/pps/pps9.pdf mentions:Hmm, is that 54, or more likely 5 and 4?
The automorphism group of the extended Golay code is the 54-transitive Mathieu group . This is one of only two finite 5-transitive groups other than symmetric and alternating groups
scite.ai/reports/4-homogeneous-groups-EAKY21 quotes link.springer.com/article/10.1007%2FBF01111290 which suggests that is is also another one of the Mathieu groups, math.stackexchange.com/questions/698327/classification-of-triply-transitive-finite-groups#comment7650505_3721840 and en.wikipedia.org/wiki/Mathieu_group_M12 mentions .
Unlisted articles are being shown, click here to show only listed articles.