Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Motivation: university sucks real bad right now Created 2024-09-06 Updated 2025-07-16
The ultimate goal: create an university:
- without entry exams
- without course requirements
- where all material is free and available online: lecture notes, problem sheets, past exam papers
- where you only pay to take certification exams for the courses that you care about
The technical goal:
Get university students to write what they learn. All university material should be amazing and free!
The how:
Create the ultimate personal knowledge base software with multi-user mind-melding features.
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects I'm not a professional hacker, I did some very occasional OSINT just for fun Created 2024-09-06 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Creative Commons CC By-SA Created 2024-09-06 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Ordinal ruleset inscription (2022): the end of the line: Eternal September arrives Created 2024-09-06 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects How illegal does something in the Bitcoin blockchain have to be to make it illegal? Created 2024-09-06 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Help wanted! Some sites were almost certainly missed. Contributions will be acknowledged. Created 2024-09-06 Updated 2025-07-16
_Britons_(Kitchener)_wants_you_(Briten_Kitchener_braucht_Euch)._1914_(Nachdruck)%2C_74_x_50_cm._(Slg.Nr._552).jpg/401px-thumbnail.jpg)
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Methodology Created 2024-09-06 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Coolest findings Created 2024-09-06 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Collateral freedom: HTTPS: the censor doesn't know which path you access Created 2024-09-06 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Xi Jinping, sadomasochist in leather suit Created 2024-09-06 Updated 2025-07-16
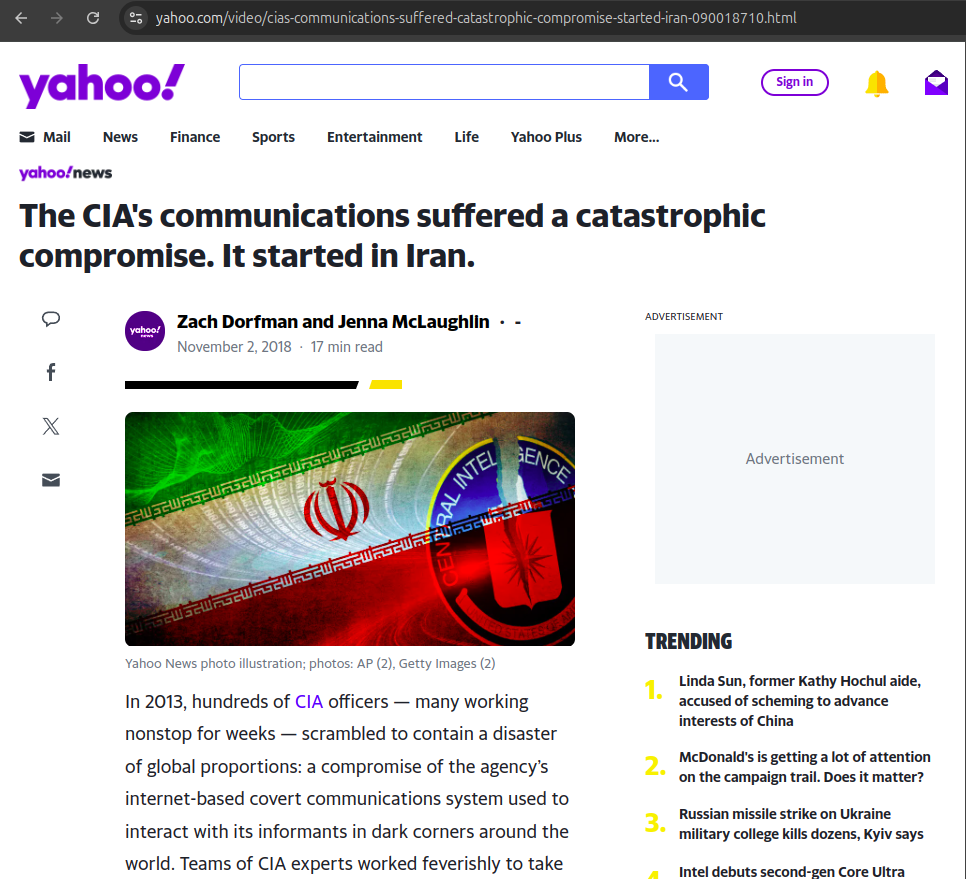
Xi Jinping, ruler of China, wearing leather sadomasochist outfit
. Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Xi Jinping, ruler of China Created 2024-09-06 Updated 2025-07-16
Xi Jinping, ruler of China
. Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Introduction Created 2024-09-06 Updated 2025-07-16
Lancelet Created 2024-09-04 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Wayback Machine searches for the communication method paths: Tor army parallelization! Created 2024-09-04 Updated 2025-07-16
com,capture-nature)/robots.txt 20211229130524 https://www.capture-nature.com/robots.txt warc/revisit - XWX2XVEZVSVIUKYXF3AJUYIRDOLOXLTO 1213
com,capture-nature)/robots.txt 20211230151913 http://capture-nature.com/robots.txt warc/revisit - XWX2XVEZVSVIUKYXF3AJUYIRDOLOXLTO 1186
com,capture-nature)/robots.txt 20220419233721 https://www.capture-nature.com/robots.txt warc/revisit - XWX2XVEZVSVIUKYXF3AJUYIRDOLOXLTO 1075
com,capture-nature)/scenes.jar 20110201104851 http://capture-nature.com/Scenes.jar application/java-archive 200 U3GPB3SPISZKLFGUJFD34C5GXWAAC2GJ 287887
com,capture-nature)/scenes.jar 20110224193204 http://capture-nature.com/Scenes.jar application/java-archive 200 U3GPB3SPISZKLFGUJFD34C5GXWAAC2GJ 287890
com,capture-nature)/scenes.jar 20130903003254 http://capture-nature.com/Scenes.jar application/x-java-archive 200 U3GPB3SPISZKLFGUJFD34C5GXWAAC2GJ 287898
com,capture-nature)/trees-and-details 20200928184446 https://www.capture-nature.com/trees-and-details text/html 200 NO6J7567VFWZLRSKBJ5HVXGT27MX2A4K 30902
com,capture-nature)/trees-and-details 20210127132910 https://www.capture-nature.com/trees-and-details text/html 200 SI73WNJUBGTOXSTRK4IRU4D4AJ637F6A 31041
com,capture-nature)/trees-and-details 20210419062751 https://www.capture-nature.com/trees-and-details text/html 200 K4Q444QJ243HW3ECXNNOBNUFMXWAPVFD 31464
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Infinitely deep table of contents Created 2024-09-04 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Publish from local markup files Created 2024-09-04 Updated 2025-07-16
- cirosantilli.com (static)
- ourbigbook.com/cirosantilli (dynamic)
Visual Studio Code extension installation
. 
Visual Studio Code extension tree navigation
. Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Web editor with side by side preview Created 2024-09-04 Updated 2025-07-16
Web editor
. You can also edit articles on the Web editor without installing anything locally. Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects My obsession: find every image before ordinals Created 2024-09-04 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Political memes? Created 2024-09-04 Updated 2025-07-16
Aratu Week 2024 Talk by Ciro Santilli: My Best Random Projects Nuclear weapon designs? Created 2024-09-04 Updated 2025-07-16
There are unlisted articles, also show them or only show them.