- phaser/hello.html: a minimal hello world adapted from web.archive.org/web/20230323212804/https://phaser.io/tutorials/getting-started-phaser3/part5. Not an actual game strictly speaking though, just shows the phaser logo bouncing around the screen.
- phaser/hello-game.html: an actually hello world game where you have to collect stars and avoid bombs.Based on labs.phaser.io/index.html?dir=games/firstgame/&q=:
- finished version: labs.phaser.io/view.html?src=src/games/firstgame/part10.js
- corresponding tutorial: web.archive.org/web/20230323210501/https://phaser.io/tutorials/making-your-first-phaser-3-game/part10.
This is Ciro Santilli's ideal university system. It is a system that actually lives up to the name "Open University":
- no enrolment, no prerequisites. Exam as a service examination style, likely free to anyone who wants to take them, only to determine:
- who gets to use physical facilities, notably laboratories
- which students do you want to pick as apprentices/workers/PhDs
- no tuition fees: free gifted education
- school must offer free accommodation for students
- force teachers to publish their teaching material with an open license
- how to teach
Related:
- reallyopenuniversity.wordpress.com/what-is-the-rou/
Apparently Leeds based. Focused only on student fees seemingly, not how to solve it with tech/efficiency:
So far as of the early 2020's, the university that comes closest to some but not all of these principles is the University of the People. It's sad that it's such a crappy unknown thing, but it is what it is.
The matrix ring of degree n is the set of all n-by-n square matrices together with the usual vector space and matrix multiplication operations.
web.archive.org/web/20181119214326/https://www.bipm.org/utils/common/pdf/CGPM-2018/26th-CGPM-Resolutions.pdf gives it in raw:The breakdown is:
- the unperturbed ground state hyperfine transition frequency of the caesium-133 atom is 9 192 631 770 Hz
- the speed of light in vacuum c is 299 792 458 m/s
- the Planck constant h is 6.626 070 15 × J s
- the elementary charge e is 1.602 176 634 × C
- the Boltzmann constant k is 1.380 649 × J/K
- the Avogadro constant NA is 6.022 140 76 × mol
- the luminous efficacy of monochromatic radiation of frequency 540 × 1012 Hz, Kcd, is 683 lm/W,
- actually use some physical constant:
the unperturbed ground state hyperfine transition frequency of the caesium-133 atom is 9 192 631 770 Hz
Defines the second in terms of caesium-133 experiments. The beauty of this definition is that we only have to count an integer number of discrete events, which is what allows us to make things precise.the speed of light in vacuum c is 299 792 458 m/s
Defines the meter in terms of speed of light experiments. We already had the second from the previous definition.the Planck constant h is 6.626 070 15 × J s
the elementary charge e is 1.602 176 634 × C
- arbitrary definitions based on the above just to match historical values as well as possible:
the Boltzmann constant k is 1.380 649 × J/K
the Avogadro constant NA is 6.022 140 76 × mol
the luminous efficacy of monochromatic radiation of frequency 540 × 1012 Hz, Kcd, is 683 lm/W
Ionization refers to the process in which atoms or molecules gain or lose electrons, resulting in the formation of charged particles known as ions. This can occur through various mechanisms, including: 1. **Loss of Electrons (Cation Formation)**: When an atom or molecule loses one or more electrons, it becomes positively charged, forming a cation. This often occurs in chemical reactions, where an atom donates an electron to another atom.
Pinned article: Introduction to the OurBigBook Project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
Intro to OurBigBook
. Source. We have two killer features:
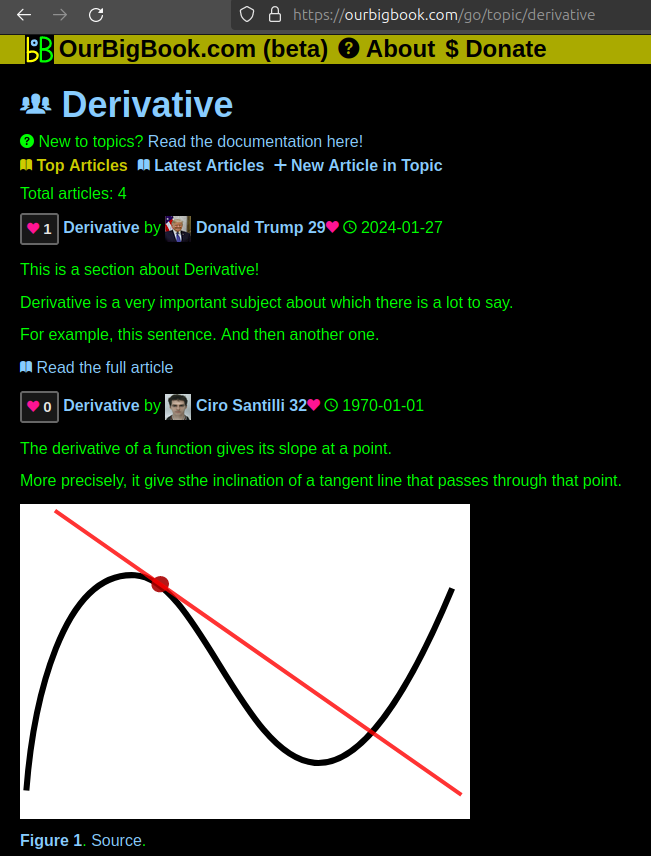
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivativeVideo 2. OurBigBook Web topics demo. Source. - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
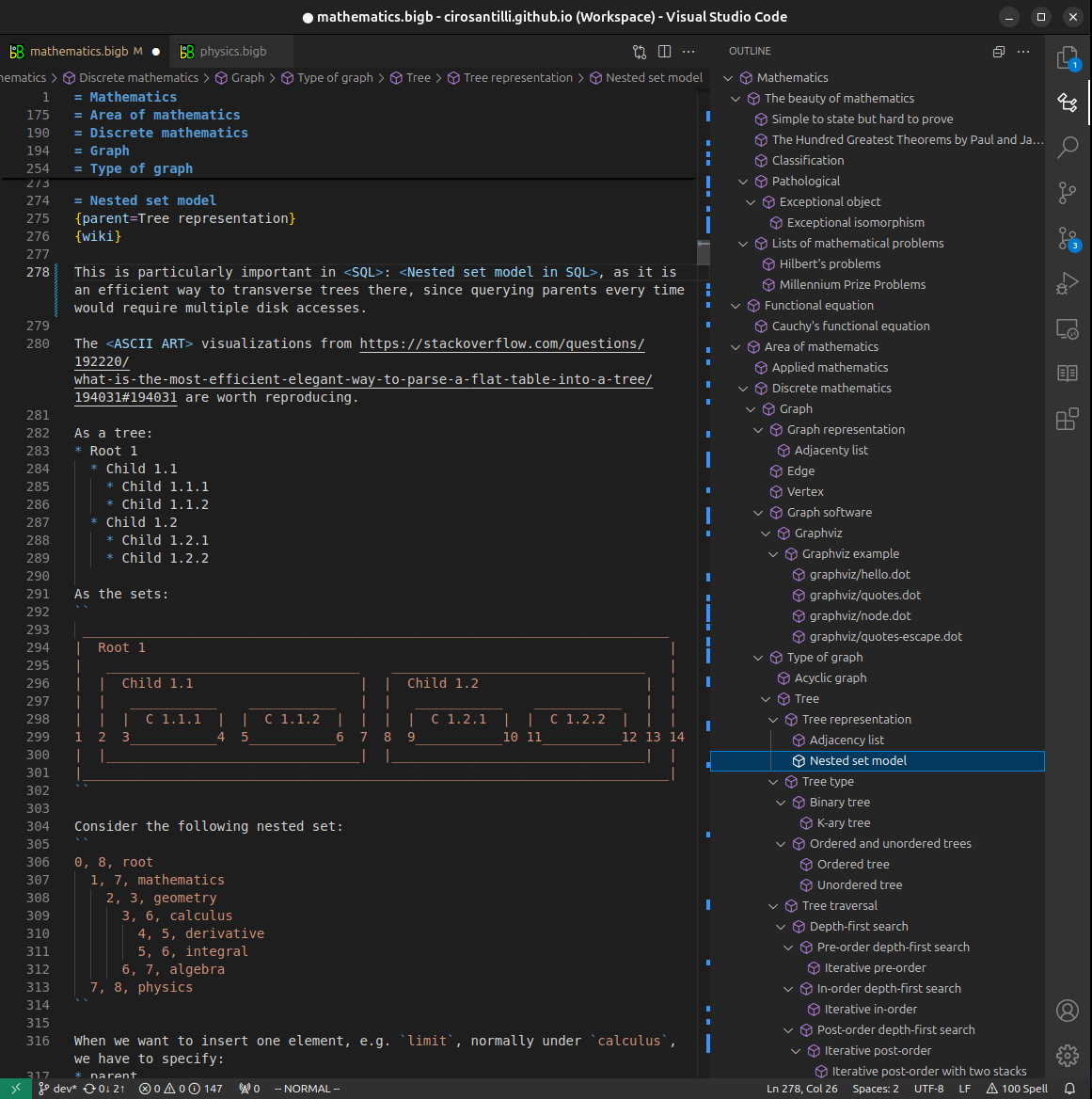
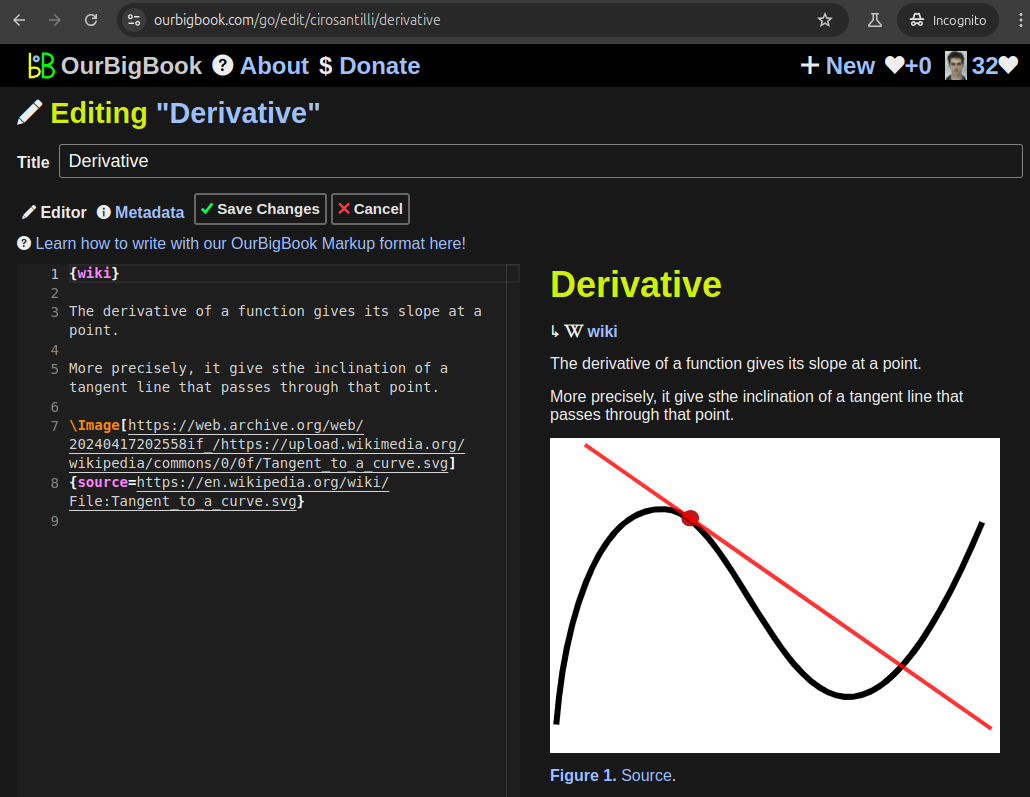
Figure 2. You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website.Figure 3. Visual Studio Code extension installation.Figure 5. . You can also edit articles on the Web editor without installing anything locally. Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension. - Infinitely deep tables of contents:
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact