It is said that you leave Oxford with either
University of Oxford student culture by 
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01 Ciro Santilli's hardware Nokia G22 (2023) by Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01£112.99
Buying October 2023 as an immediate backup phone after the Google Pixel 3a (2020) touchscreen died, and Motorola Moto G6 Play (2018) wouldn't connect to giffgaff.
Still working checks: May 2024.
Ciro Santilli's hardware House 2018-01 Toilet mirror lamps by Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01When I came, two Bell GU4 (MR11) 20W 12v.
One burnt. Put in an ASDA halogen one.
ASDA burnt, put in TopLux on right, old Bell left.
2019-01-24, right one burnt a few days ago, old Bell still works. Inner part black, and black dot on the wire. Putting new TopLux again, but this time on the left, old bell on right.
2019-01-24 toilet top lamp also burnt a few days ago, but not at the same time as mirror. Diall, 240V 40W, GU10. Putting in IKEA 240V 35W.
2019-02-02 toilet mirror lamp left (TopLux) burnt. Don't know what to do anymore. Only the magic Bell lamp works.
2019-03-06 toilet top lamp left burnt, IKEA 240V 35W GU10. Putting in another one.
2019-03-28 toilet top lamp right burnt, IKEA 240V 35W GU10. Waiting for people to come to look at transformer, there is definitely something wrong.
2019-04-03 top lamps: replaced with LED (LAP GU10 3W) since lower power, transformer not changed. Mirror lamps: transformer changed, left one replaced with Homebase Halogen 20W 12V. When I came back lamps flickering badly and sometimes not turning on, recalled technician.
2019-04-12 mirror lamp: it was just he connector that was bad, it was changed, also put LEDs there to make it less warm and hopefully have less tear on connector.
Ciro Santilli's hardware Park Tool PW-5 (2022) by Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01Bought: 2022.
To remove pedals when they get stiff.
Introductory Quantum Mechanics by Richard Fitzpatrick (2020) by Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01This LibreTexts book does have some interest!
It is hard to decide what makes Ciro Santilli more sad: the usage of Greek letters, the invention of new symbols, or the fifty million alternative font styles used.
Only Chinese characters could be worse than that!
Ciro Santilli's hardware House 2018-01 Mould by Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01 Ciro Santilli's hardware House 2018-01 by Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01 Ciro Santilli's hardware Prestige 7.5L Stainless Stell Pressure Cooker (2020) by Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01Primarily bought to make borlotti beans!!!
Ciro Santilli's hardware Miele C2 Complete PowerLine vacuum cleaner by Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01
Ciro Santilli 37 Updated 2025-05-09 +Created 1970-01-01Similar to: www.miele.co.uk/e/cylinder-vacuum-cleaner-compact-c2-powerline-sdrf4-10931670-p but slightly different from image. Indistinguishable from: www.currys.co.uk/gbuk/home-appliances/floorcare/vacuum-cleaners/miele-complete-c2-pure-power-powerline-cylinder-vacuum-cleaner-graphite-grey-10208570-pdt.html
Power symbol meanings: images-eu.ssl-images-amazon.com/images/I/A14OZ5TuwGS.pdf
2020-12: buying Miele SF AP 50 AirClean Plus filter www.miele.co.uk/e/airclean-plus-filter-sf-ap-50-10107860-p. Once it arrived, changed the filter, which fit comes with plastic casing and accordion shaped filter. Also changed bag. But the bag full meter still goes to red on carpet even with everything new while vacuuming. Is it supposed to be checked while not vacuuming?
There are 5: en.wikipedia.org/w/index.php?title=Euclidean_geometry&oldid=1036511366#Axioms, the parallel postulate being the most controversial/interesting.
Pinned article: ourbigbook/introduction-to-the-ourbigbook-project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
Intro to OurBigBook
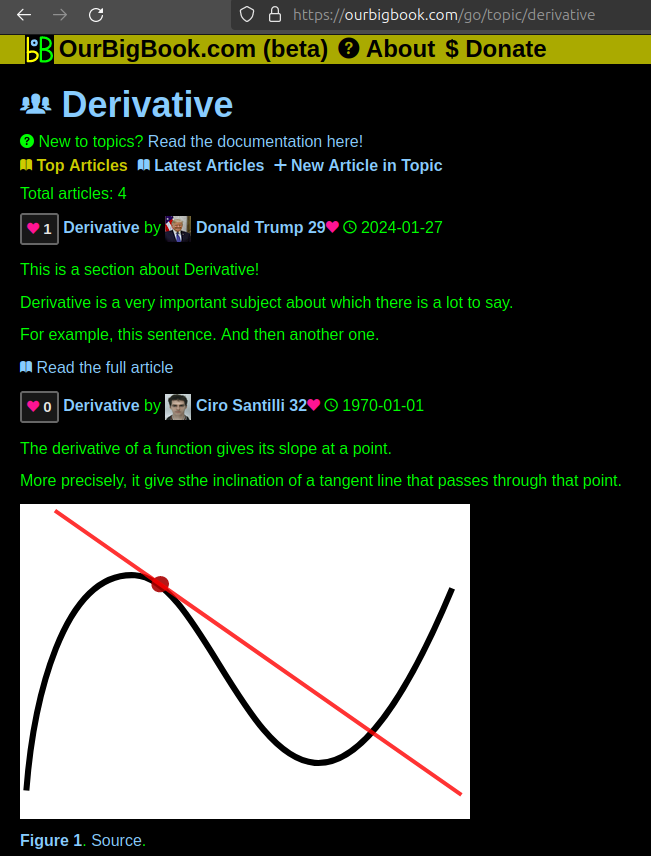
. Source. We have two killer features:
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivativeVideo 2. OurBigBook Web topics demo. Source. - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
Figure 2. You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website.
Figure 3. Visual Studio Code extension installation.
Figure 5. . You can also edit articles on the Web editor without installing anything locally. Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension. 
- Infinitely deep tables of contents:
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact