There are two cases:
- (topological) manifolds
- differential manifolds
Questions: are all compact manifolds / differential manifolds homotopic / diffeomorphic to the sphere in that dimension?
- for topological manifolds: this is a generalization of the Poincaré conjecture.Original problem posed, for topological manifolds.Last to be proven, only the 4-differential manifold case missing as of 2013.Even the truth for all was proven in the 60's!Why is low dimension harder than high dimension?? Surprise!AKA: classification of compact 3-manifolds. The result turned out to be even simpler than compact 2-manifolds: there is only one, and it is equal to the 3-sphere.For dimension two, we know there are infinitely many: classification of closed surfaces
- for differential manifolds:Not true in general. First counter example is . Surprise: what is special about the number 7!?Counter examples are called exotic spheres.Totally unpredictable count table:
Dimension Smooth types 1 1 2 1 3 1 4 ? 5 1 6 1 7 28 8 2 9 8 10 6 11 992 12 1 13 3 14 2 15 16256 16 2 17 16 18 16 19 523264 20 24 is an open problem, there could even be infinitely many. Again, why are things more complicated in lower dimensions??
Mathematical consistency of quantum field theory by 
Ciro Santilli 35 Updated 2025-03-28 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-03-28 +Created 1970-01-01It is hard to say if this channel is good because of the awesome information, or if because of the absolute cutness of that British presenter. Maybe it is both.
Communicating at a distance, from Greek "tele" for distance!
A very cool thing about telecommunication is, besides how incredibly fast it advanced (in this sense it is no cooler than integrated circuit development), how much physics and information theory is involved in it. Applications of telecommunication implementation spill over to other fields, e.g. some proposed quantum computing approaches are remarkably related to telecommunication technology, e.g. microwaves and silicon photonics.
This understanding made Ciro Santilli wish he had opted for telecommunication engineering when he was back in school in Brazil. For some incomprehensible reason, telecommunications was the least competitive specialization in the electric engineering department at the time, behind even power electronics. This goes to show both how completely unrelated to reality university is, and how completely outdated Brazil is/was. Sad stuff.
Testing and Circuit for a Condenser microphone by RSD Academy (2018)
Source. Not very numerical, but shows a simple working breadboard circuit and an oscilloscope. He whistles with his mouth to get a pretty pure frequency.
That type of microphone requires a bias voltage. The circuit is in Ciro's ASCII art circuit diagram notation:
DC_9---R_10k--+--MICROPHONE--+--G
| |
+-------V------+Soundwaves on an oscilloscope by Animated Science (2015)
Source. Dude speaking to microphone. Some analysis of how different sounds look like. No circuit diagram. Pinned article: ourbigbook/introduction-to-the-ourbigbook-project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
We have two killer features:
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
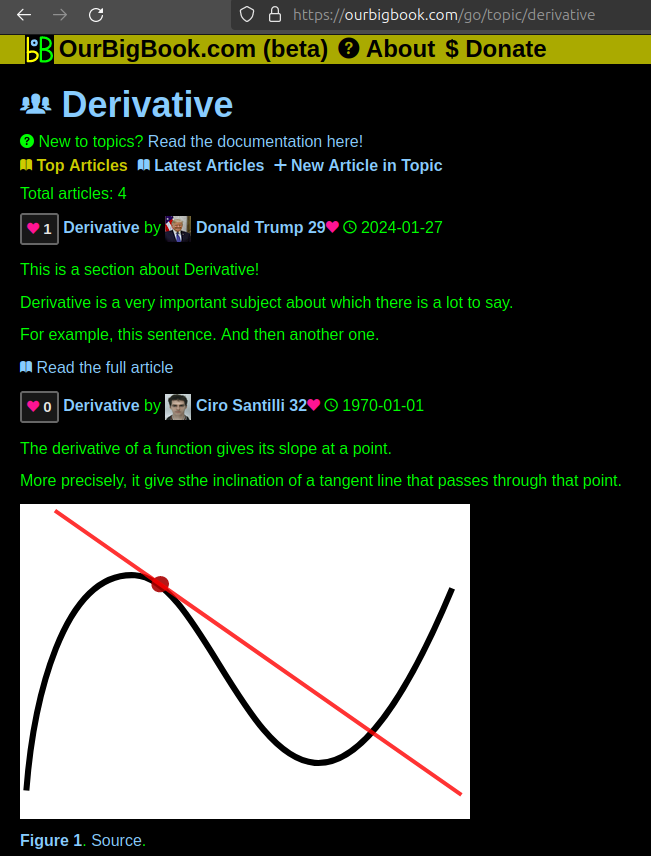
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivative - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
Figure 2. You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website.
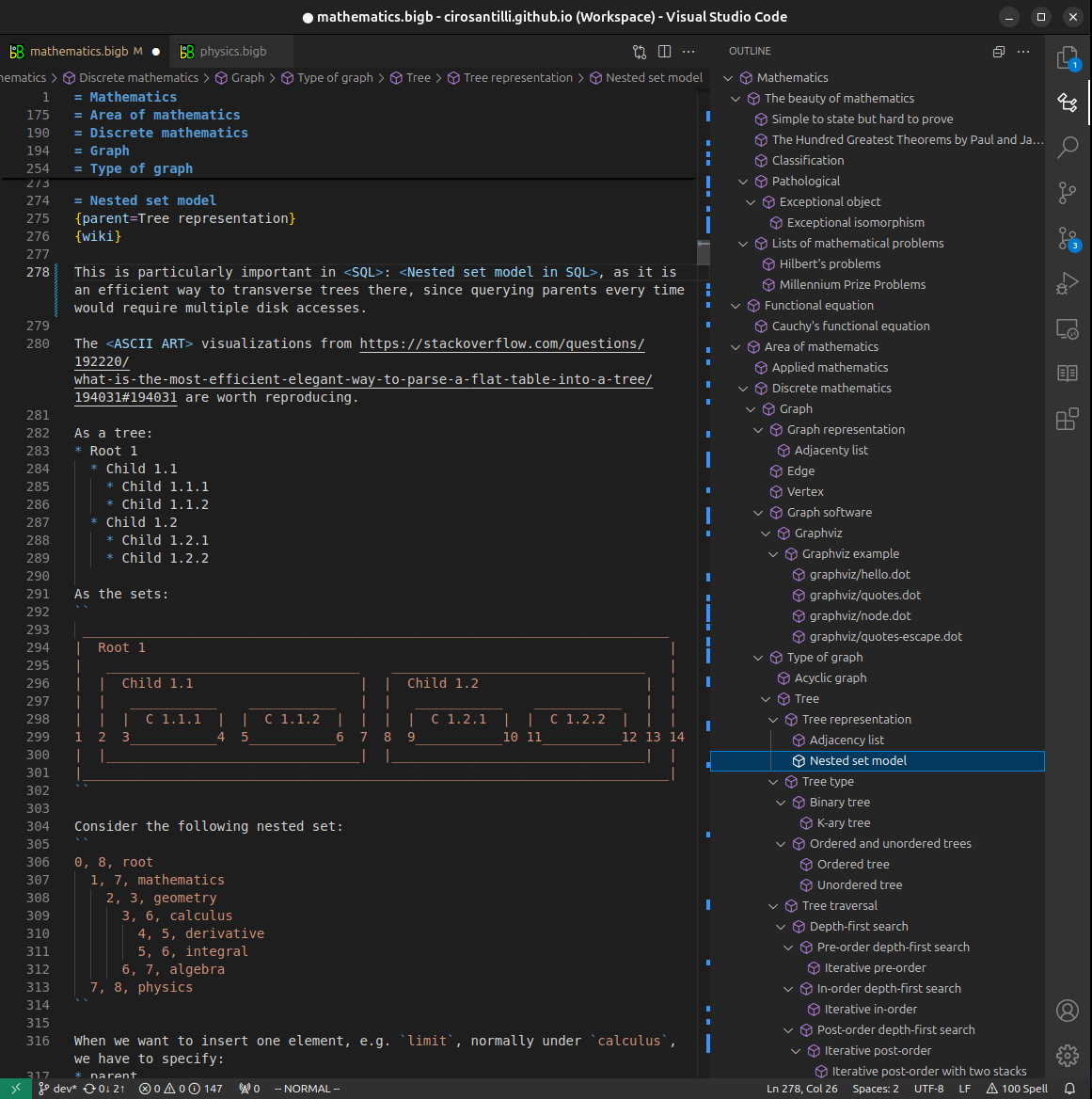
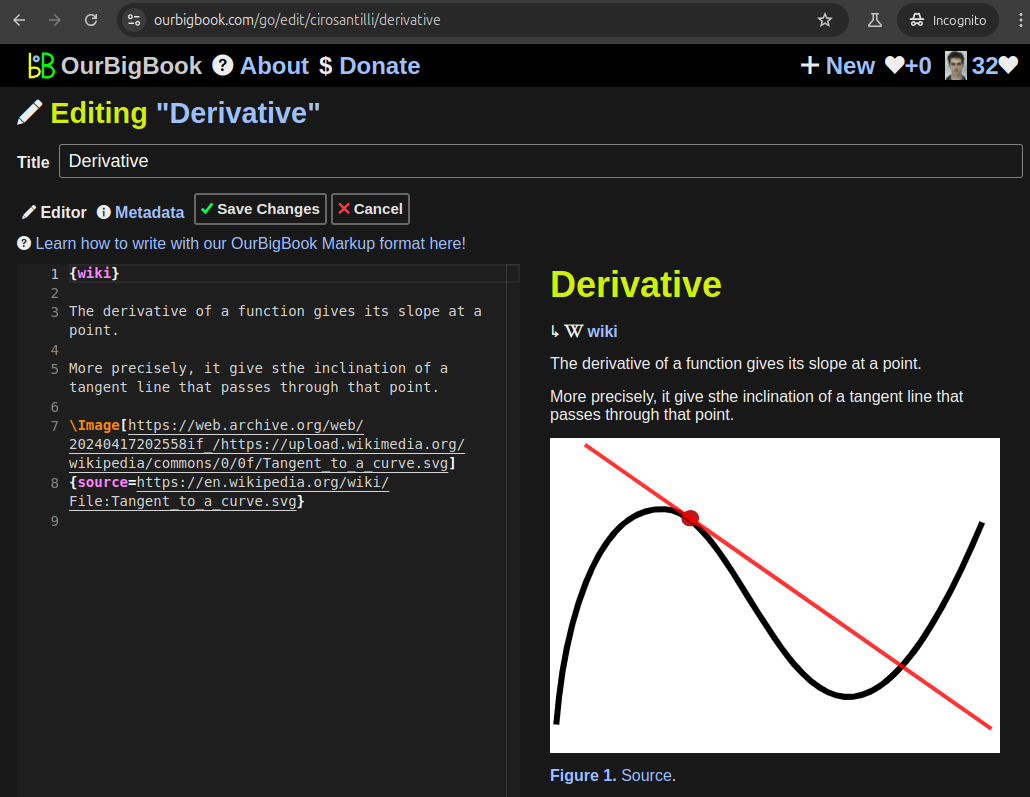
Figure 3. Visual Studio Code extension installation.
Figure 4. Visual Studio Code extension tree navigation.
Figure 5. . You can also edit articles on the Web editor without installing anything locally. Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension.Video 4. OurBigBook Visual Studio Code extension editing and navigation demo. Source. - Internal cross file references done right:
- Infinitely deep tables of contents:
Figure 6. Dynamic article tree with infinitely deep table of contents.Live URL: ourbigbook.com/cirosantilli/chordateDescendant pages can also show up as toplevel e.g.: ourbigbook.com/cirosantilli/chordate-subclade
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact