Each elliptic space can be modelled with a real projective space. The best thing is to just start thinking about the real projective plane.
Generalize function to allow adding some useful things which people wanted to be classical functions but which are not,
It therefore requires you to redefine and reprove all of calculus.
For this reason, most people are tempted to assume that all the hand wavy intuitive arguments undergrad teachers give are true and just move on with life. And they generally are.
One notable example where distributions pop up are the eigenvectors of the position operator in quantum mechanics, which are given by Dirac delta functions, which is most commonly rigorously defined in terms of distribution.
Distributions are also defined in a way that allows you to do calculus on them. Notably, you can define a derivative, and the derivative of the Heaviside step function is the Dirac delta function.
There's not way to describe it as a classical function, making it the most important example of a distribution.
Applications:
- position operator in quantum mechanics. It's not a coincidence that the function is named after Paul Dirac.
Being a complex holomorphic function is an extremely strong condition.
Another extremely strong consequence is the identity theorem.
visualizing the Riemann hypothesis and analytic continuation by 3Blue1Brown (2016) is a good quick visual non-mathematical introduction is to it.
The key question is: how can this continuation be unique since we are defining the function outside of its original domain?
The answer is: due to the identity theorem.
Pinned article: Introduction to the OurBigBook Project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
Intro to OurBigBook
. Source. We have two killer features:
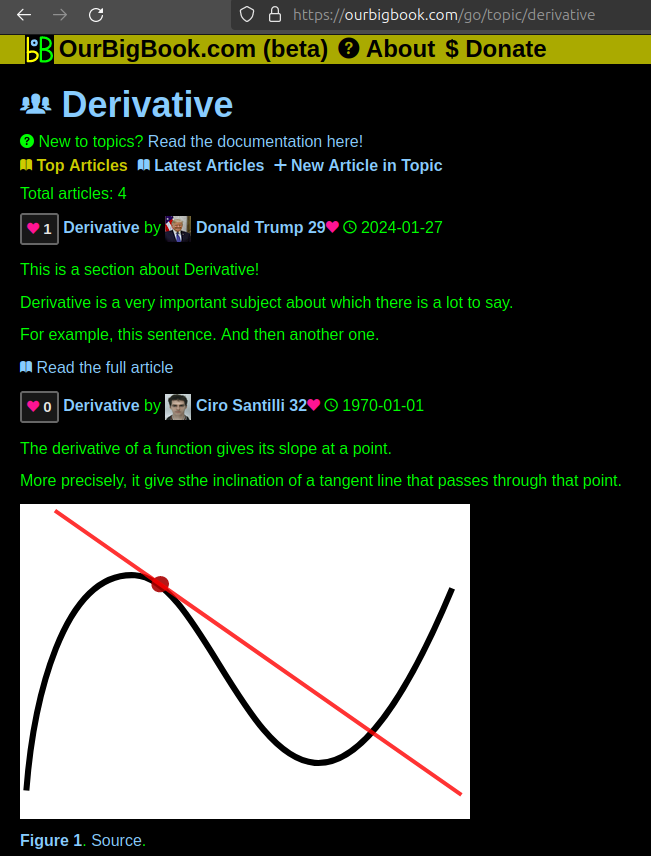
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivativeVideo 2. OurBigBook Web topics demo. Source. - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
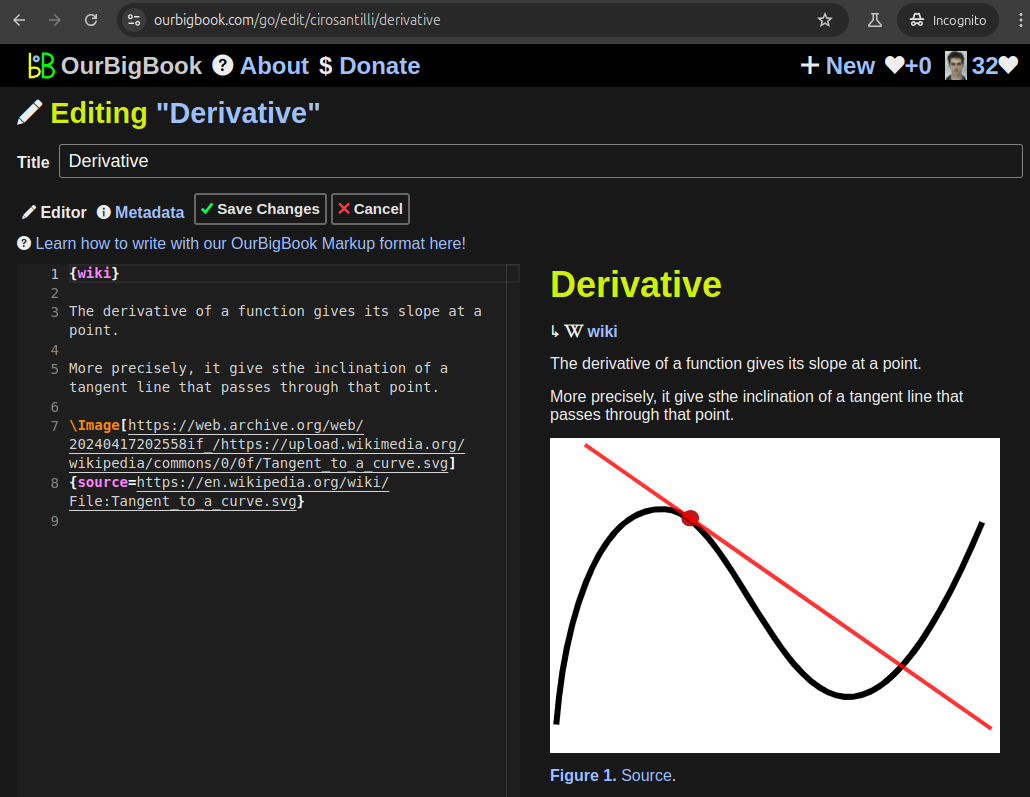
Figure 3. Visual Studio Code extension installation.
Figure 4. Visual Studio Code extension tree navigation.
Figure 5. Web editor. You can also edit articles on the Web editor without installing anything locally.Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension.Video 4. OurBigBook Visual Studio Code extension editing and navigation demo. Source. 
- Infinitely deep tables of contents:
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact