MATLAB Breaking Bad crossover
. Source. 
By "Satoshi uploader" we mean the data upload script present in tx 4b72a223007eab8a951d43edc171befeabc7b5dca4213770c88e09ba5b936e17 of the Bitcoin blockchain.
The uploader, and its accompanying downloader, are Python programs stored in the blockchain itself. They are made to upload and download arbitrary data into the blockchain via RPC.
These scripts were notably used for: illegal content of block 229k. The script did not maintain its popularity much after this initial surge up loads, likely all done by the same user: there are very very few uploads done after block 229k with the Satoshi uploader.
Our choice of name as "Satoshi uploader" is copied from A Quantitative Analysis of the Impact of Arbitrary Blockchain Content on Bitcoin by Matzutt et al. (2018) because the scripts are Copyrighted Satoshi Nakamoto on the header comment, although as mentioned at Hidden surprises in the Bitcoin blockchain by Ken Shirriff (2014) this feels very unlikely to be true.
A more convenient version of those scripts that can download directly from blockchain.info without the need for a full local node can be found at: github.com/cirosantilli/bitcoin-inscription-indexer/blob/master/download_tx_consts.py by using the
--satoshi option. E.g. with it you can download the uploader script with:./download_tx_consts.py --satoshi 4b72a223007eab8a951d43edc171befeabc7b5dca4213770c88e09ba5b936e17
mv 4b72a223007eab8a951d43edc171befeabc7b5dca4213770c88e09ba5b936e17.bin uploader.pyThe scripts can be found in the blockchain at:
- uploader: tx 4b72a223007eab8a951d43edc171befeabc7b5dca4213770c88e09ba5b936e17 block 229991 reproduced at: gist.github.com/cirosantilli/ade4dde7c2f2f5020d792872681763e8The uploader creates a standard Pay-to-PubkeyHash transaction with a single output and data as a fake pubkey hash, and sends change to an address specified on the command line:
./bitcoinInsertionTool.py <data> <change-addr> - downloader: tx 6c53cd987119ef797d5adccd76241247988a0a5ef783572a9972e7371c5fb0cc block 229991 reproduced at gist.github.com/cirosantilli/e90bd2e6c3fab25a20898e61e3ab3e90The downloader just strips all operands, and keeps all data, notably where public key hashes would be normally put.
The uploader script uses its own cumbersome data encoding format, which we call the "Satoshi uploader format". The is as follows:This means that if we want to index certain file types encoded in this format, a good heuristic is to skip the first 9 bytes (4 size, 4 CRC, 1
- ignore all script operands and constants less than 20 bytes (40 hex characters). And there are a lot of small operands, e.g. the uploader itself uses format www.blockchain.com/btc/tx/4b72a223007eab8a951d43edc171befeabc7b5dca4213770c88e09ba5b936e17 has a
OP_1, data,OP_3,OP_CHECKMULTISIGpattern on every output script, so theOP_1andOP_3are ignored. I.e., it is P2FMS. - ignore the last output, which contains a real change transaction instead of arbitrary data. TODO why not just do what with the length instead?
- the first 4 bytes are the payload length, the next 4 bytes a CRC-32 signature. The payload length is in particular useful because of possible granularity of transactions. But it is hard to understand why a CRC-32 is needed in the middle of the largest hash tree ever created by human kind!!! It does however have the adavantage that it allows us to more uniquely identify which transactions use the format or not.
OP_1) and look for file signatures.Let's try out the downloader to download itself. First you have to be running a Bitcoin Core server locally. Then, supposing we run:worked! The source of the downloader script is visible! Note that we had to wait for the sync of the entire blockchain to be fully finished for some reason for that to work.
.bitcon/bitoin.conf containing:rpcuser=asdf
rpcpassword=qwer
server=1
txindex=1git clone git://github.com/jgarzik/python-bitcoinrpc.git
git -C python-bitcoinrpc checkout cdf43b41f982b4f811cd4ebfbc787ab2abf5c94a
wget https://gist.githubusercontent.com/shirriff/64f48fa09a61b56ffcf9/raw/ad1d2e041edc0fb7ef23402e64eeb92c045b5ef7/bitcoin-file-downloader.py
pip install python-bitcoinrpc==1.0
BTCRPCURL=http://asdf:qwer@127.0.0.1:8332 \
PYTHONPATH="$(pwd)/python-bitcoinrpc:$PYTHONPATH" \
python3 bitcoin-file-downloader.py \
6c53cd987119ef797d5adccd76241247988a0a5ef783572a9972e7371c5fb0ccOther known uploads in Satoshi format except from the first few:
- tx 89248ecadd51ada613cf8bdf46c174c57842e51de4f99f4bbd8b8b34d3cb7792 block 344068 see ASCII art
- tx 1ff17021495e4afb27f2f55cc1ef487c48e33bd5a472a4a68c56a84fc38871ec contains the ASCII text
e5a6f30ff7d43f96f61af05efaf96f869aa072b5a071f32a24b03702d1dcd2a6. This number however is not a known transaction ID in the blockchain, and has no Google hits.
We map each point and a small enough neighbourhood of it to , so we can talk about the manifold points in terms of coordinates.
Does not require any further structure besides a consistent topological map. Notably, does not require metric nor an addition operation to make a vector space.
A notable example of a Non-Euclidean geometry manifold is the space of generalized coordinates of a Lagrangian. For example, in a problem such as the double pendulum, some of those generalized coordinates could be angles, which wrap around and thus are not euclidean.
Historic, unique Manhattan Project footage from Los Alamos by Los Alamos National Lab
. Source. Mostly the daily life part of things, but very good, includes subtitles explaining the people and places shown.
Marked with identifier "LA-UR 11-4449".
The input script of the Coinbase transaction can be anything, and this can be used as a Bitcoin inscription method.
Notable examples:
- Genesis block message
- Prayer side of the Prayer wars
DealBook Conference 2015 - Activist Investing by New York Times Events (2015)
Source. At this timestamp, Carl Icahn tells an anecdote of how he found that an entire 12-floor building of the American Car and Foundry Company which he had recently bought was not doing anything useful, and fired the entire building.This incredibly foul mouthed band was incredibly funny.
Ciro Santilli was a bit young to understand the songs at the time, but the older boys were singing them, and he sang along. So maybe there is a nostalgia factor in play.
But it can't be just that. They are just too funny and brutal, even when Ciro re-listens to it as of 2020.
Many Brazilian religious cranks in were really happy when their plane crashed and killed all of them in 1996.
Official YouTube channel: www.youtube.com/channel/UCPmfP5LNbxYwlN0k6C3F_6w
Best songs:
- Pelados Em Santos (1995). Not too amazing, they only try to be funny by making fun of the North-Eastern accent of Brazil, which is considered somewhat funny in São Paulo, and they fail at being funny.But it talks about Santos, São Paulo, Brazil, Ciro's idolized city, so let's hear it. They didn't live in Santos apparently, but being from São Paulo City, they would have been familiar with that popular local beach location.
Active compound in pepper.
Pinned article: ourbigbook/introduction-to-the-ourbigbook-project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
Intro to OurBigBook
. Source. We have two killer features:
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
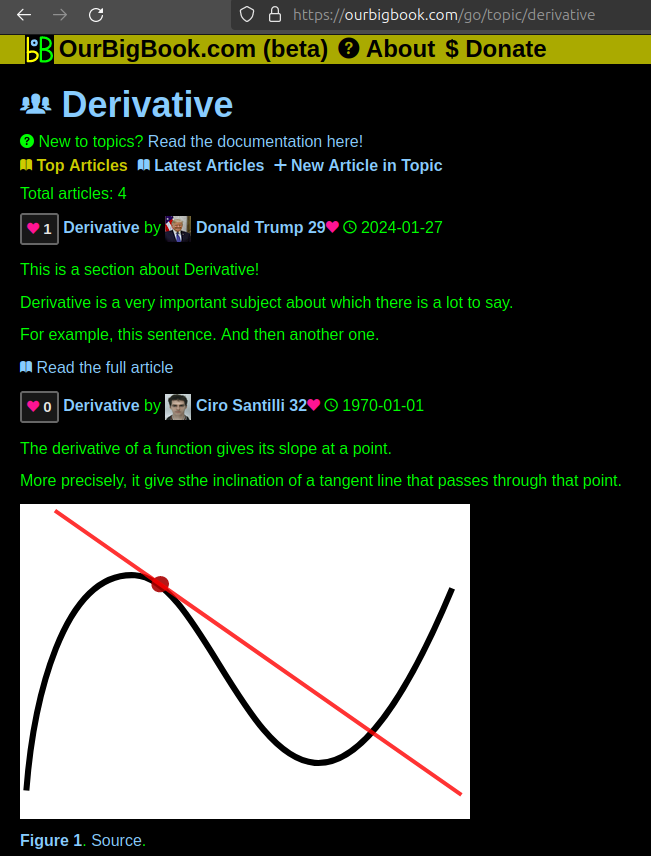
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivativeVideo 2. OurBigBook Web topics demo. Source. - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
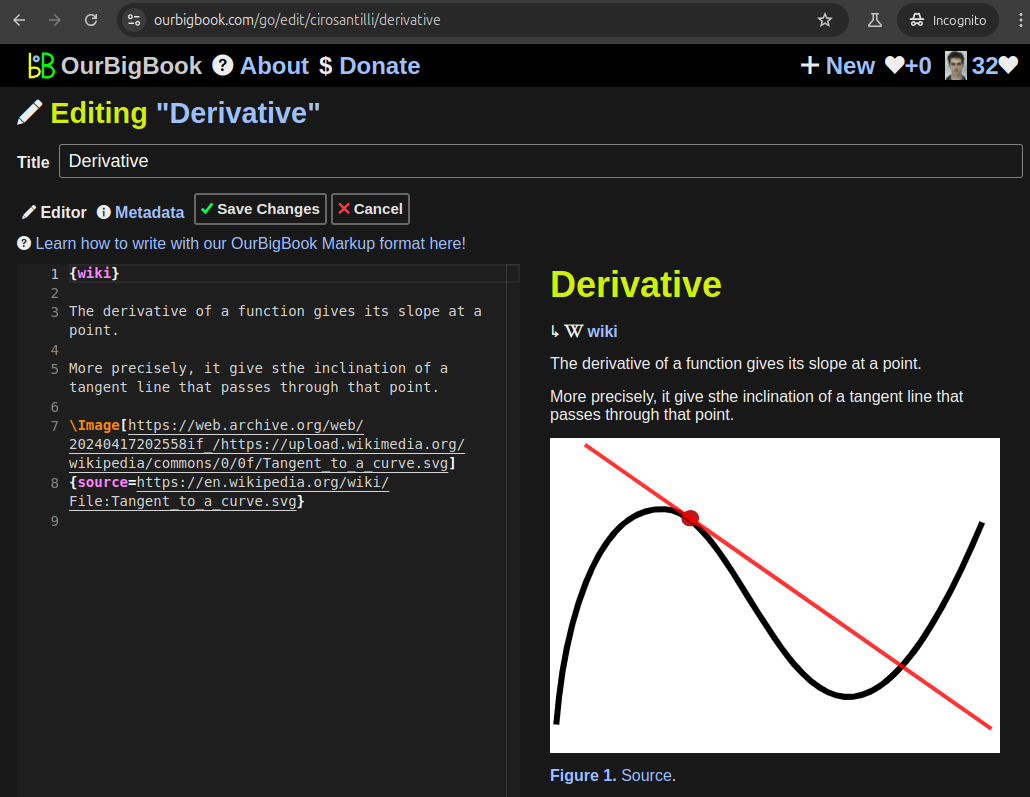
Figure 2. You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website.
Figure 3. Visual Studio Code extension installation.
Figure 5. . You can also edit articles on the Web editor without installing anything locally. Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension. 
- Infinitely deep tables of contents:
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact