But now people want to send voice. How to do it?
It would not be practical without modulation: Why can't you send voice without modulation?
Sample implementations:
One of their learning sites: www.qutube.nl/
The educational/outreach branch of QuTech.
One direct practical reason is that we need to map the matrix to real quantum hardware somehow, and all quantum hardware designs so far and likely in the future are gate-based: you manipulate a small number of qubits at a time (2) and add more and more of such operations.
While there are "quantum compilers" to increase the portability of quantum programs, it is to be expected that programs manually crafted for a specific hardware will be more efficient just like in classic computers.
TODO: is there any clear reason why computers can't beat humans in approximating any unitary matrix with a gate set?
This is analogous to what classic circuit programmers will do, by using smaller logic gates to create complex circuits, rather than directly creating one huge truth table.
The gates themselves are just unitary matrices that operate on the input qubits and produce the same number of output qubits.
For example, the matrix for the CNOT gate, which takes 2 qubits as input is:
1 0 0 0
0 1 0 0
0 0 0 1
0 0 1 0The final question is then: if I have a 2 qubit gate but an input with more qubits, say 3 qubits, then what does the 2 qubit gate (4x4 matrix) do for the final big 3 qubit matrix (8x8)? In order words, how do we scale quantum gates up to match the total number of qubits?
The intuitive answer is simple: we "just" extend the small matrix with a larger identity matrix so that the sum of the probabilities third bit is unaffected.
More precisely, we likely have to extend the matrix in a way such that the partial measurement of the original small gate qubits leaves all other qubits unaffected.
For example, if the circuit were made up of a CNOT gate operating on the first and second qubits as in:
0 ----+----- 0
|
1 ---CNOT--- 1
2 ---------- 2TODO lazy to properly learn right now. Apparently you have to use the Kronecker product by the identity matrix. Also, zX-calculus appears to provide a powerful alternative method in some/all cases.
- quantumtech.blog/2023/01/17/quantum-computing-with-neutral-atoms/ OK this one hits it:So we understand that it is truly like the classical computer analog vs digital case.
As Alex Keesling, CEO of QuEra told me, "... whereas in gate-based [digital] quantum computing the focus is on the sequence of the gates, in analog quantum processing it's more about the position of the atoms and where you place them so they can mirror real life problems. We arrange the atoms and define the forces that drive them and then measure the result... so it’s a geometric encoding of the problem itself."
- thequantuminsider.com/2022/06/28/why-analog-neutral-atoms-quantum-computing-is-a-promising-direction-for-early-quantum-advantage on The Quantum Insider useless article mostly by Pasqal
TensorFlow quantum by Masoud Mohseni (2020)
Source. At the timestamp, Masoud gives a thought experiment example of the perhaps simplest to understand analog quantum computer: chained double-slit experiments with carefully calculated distances between slits. Calulating the final propability distribution of that grows exponentially.Non-linearity is needed otherwise the input energy would just make the state go to higher and higher energy levels, e.g. from 1 to 2. But we only want to use levels 0 and 1.
The way this is modelled in by starting from a pure LC circuit, which is an harmonic oscillator, see also quantum LC circuit, and then replacing the linear inductor with a SQUID device, e.g. mentioned at: youtu.be/eZJjQGu85Ps?t=1655 Video "Superconducting Qubits I Part 1 by Zlatko Minev (2020)".
Superconducting qubits are bad because it is harder to ensure that they are all the same by 
Ciro Santilli 40 Updated 2025-07-16
Ciro Santilli 40 Updated 2025-07-16This is unlike atomic systems like trapped ion quantum computers, where each atom is necessarily exactly the same as the other.
Most of the helium in the Earth's atmosphere comes from alpha decay, since helium is lighter than air and naturally escapes out out of the atmosphere.
Wiki mentions that alpha decay is well modelled as a quantum tunnelling event, see also Geiger-Nuttall law.
As a result of that law, alpha particles have relatively little energy variation around 5 MeV or a speed of about 5% of the speed of light for any element, because the energy is inversely exponentially proportional to half-life. This is because:
- youtu.be/_f8zeEI0oys?t=796 George Gamow and Edward Condon proposed the quantum tunnelling explanation
- youtu.be/_f8zeEI0oys?t=1725 worked out example that predicts the half-life of polonium-210 based on its emission energy
None known as of 2020.
Pinned article: Introduction to the OurBigBook Project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
Intro to OurBigBook
. Source. We have two killer features:
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
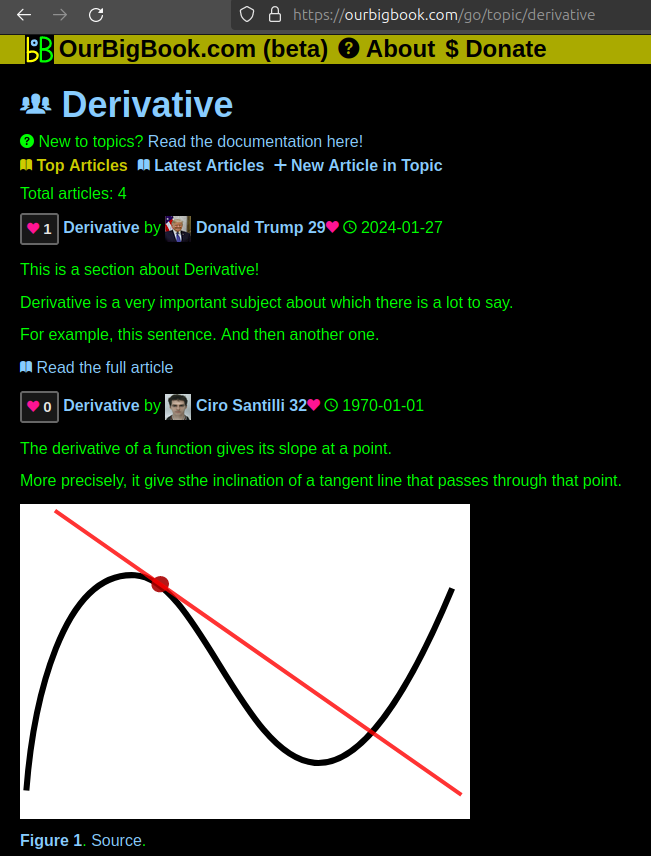
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivativeVideo 2. OurBigBook Web topics demo. Source. - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
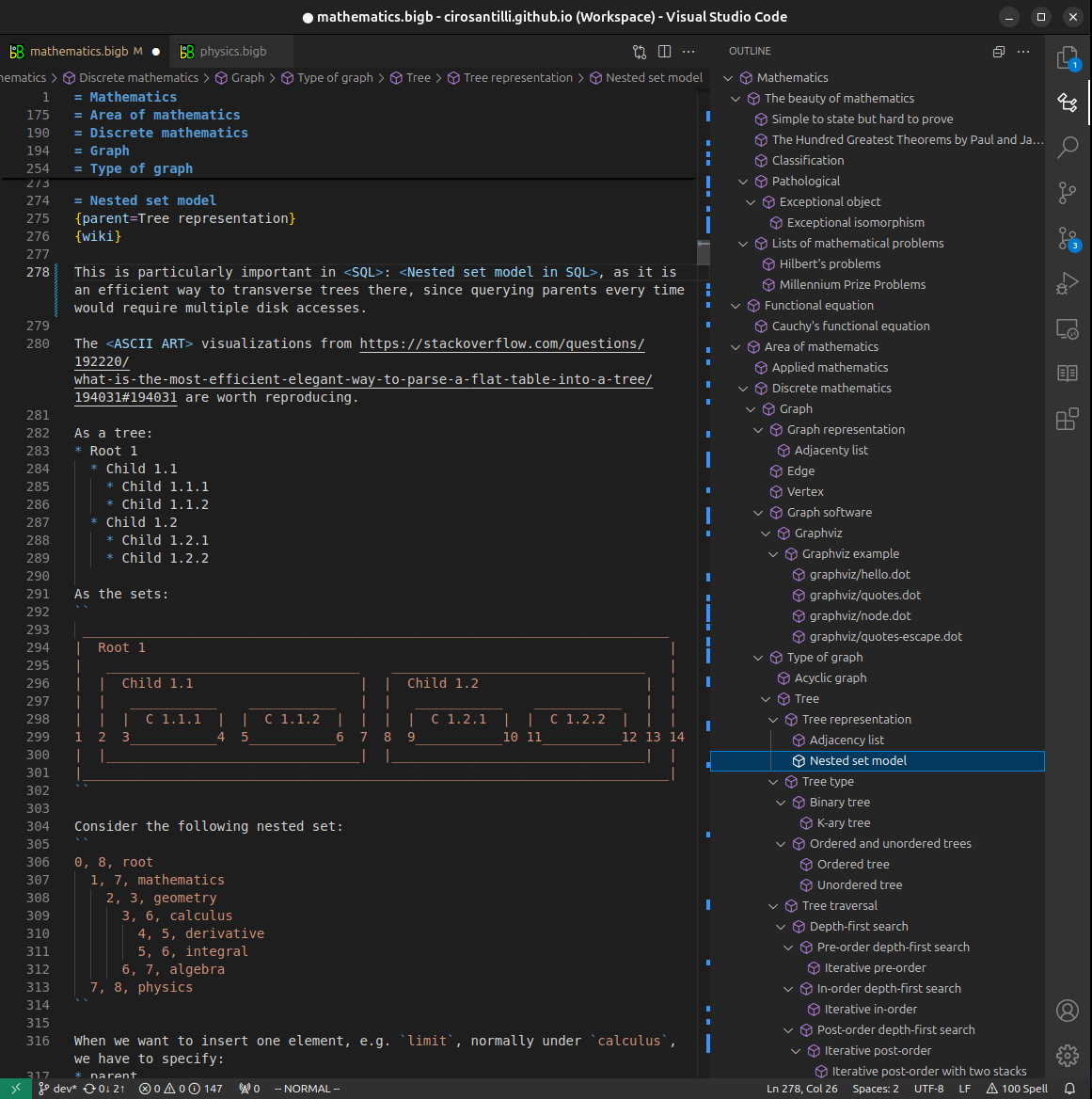
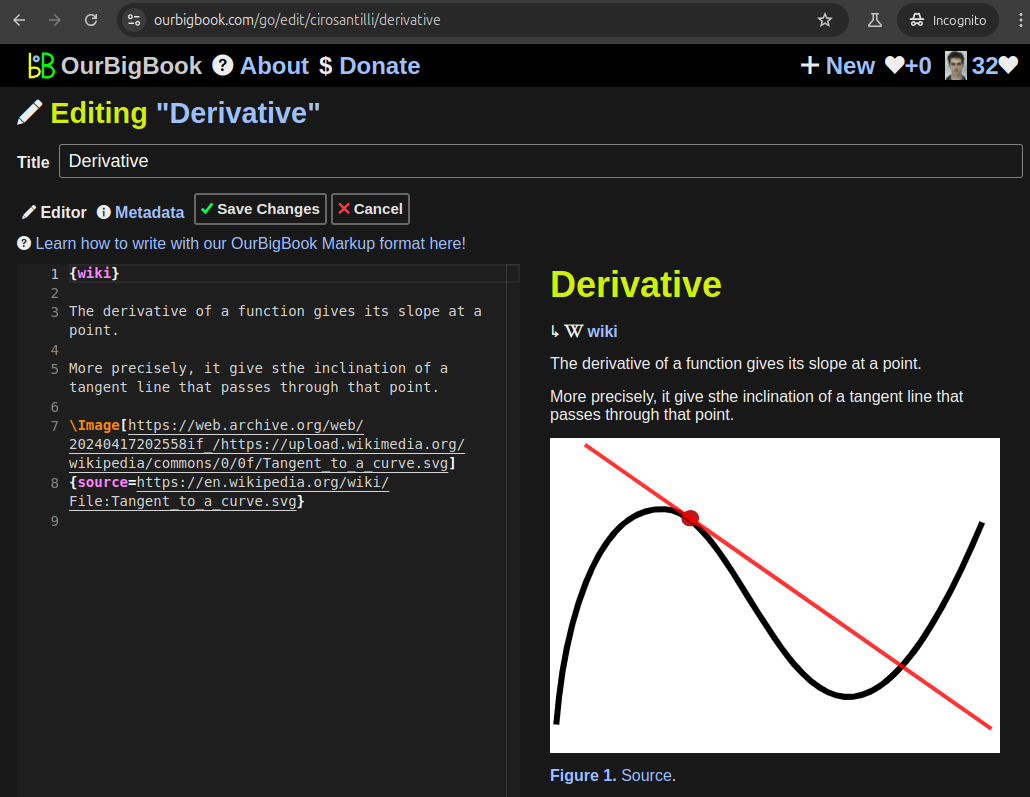
Figure 3. Visual Studio Code extension installation.
Figure 4. Visual Studio Code extension tree navigation.
Figure 5. Web editor. You can also edit articles on the Web editor without installing anything locally.Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension.Video 4. OurBigBook Visual Studio Code extension editing and navigation demo. Source. 
- Infinitely deep tables of contents:
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact