All groups are isomorphic to a subgroup of the symmetric group by 
Ciro Santilli 35 Updated 2025-03-28 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-03-28 +Created 1970-01-01Or in other words: symmetric groups are boring, because they are basically everything already!
By default, NASM places a
.symtab on the executable as well.This is only used for debugging. Without the symbols, we are completely blind, and must reverse engineer everything.
You can strip it with
objcopy, and the executable will still run. Such executables are called "stripped executables".The inverse of the magnetic flux quantum.
17 of them.
Bought December 2023 for 300 pounds, so 15 pounds / TB. Needs power supply unfrotunately besides USB, the largest one without needing power supply was only 5 TB at the time so not worth it, given that my P14s alreay carriers 2 TB. 10x is a must for the external crap.
www.westerndigital.com/en-gb/products/external-drives/wd-elements-desktop-usb-3-0-hdd?sku=WDBWLG0030HBK-EESN (archive)
Noise: it has a constant pleasant hum, with a not so nice click every 5 seconds or so. Reasonable, but not amazingly incredible.
The size is reasonable, not megaportable, but definitely reasonable.
Holds strings for the symbol table.
This section has
sh_type == SHT_STRTAB.It is pointed to by outputs:
sh_link == 5 of the .symtab section.readelf -x .strtab hello_world.oHex dump of section '.strtab':
0x00000000 0068656c 6c6f5f77 6f726c64 2e61736d .hello_world.asm
0x00000010 0068656c 6c6f5f77 6f726c64 0068656c .hello_world.hel
0x00000020 6c6f5f77 6f726c64 5f6c656e 005f7374 lo_world_len._st
0x00000030 61727400 art.This implies that it is an ELF level limitation that global variables cannot contain NUL characters.
~1TB.
Internal hard drive likely removed from some old computer I lost track of, kept in a crappy case, incredible stuff.
Ubuntu 20.04
gnome-disks benchmark, NTFS partition: 40MB/s.Besides
sh_type == SHT_RELA, there also exists SHT_REL, which would have section name .text.rel (not present in this object file).Those represent the same
struct, but without the addend, e.g.:typedef struct {
Elf64_Addr r_offset;
Elf64_Xword r_info;
} Elf64_Rela;The ELF standard says that in many cases the both can be used, and it is just a matter of convenience.
This program did not have certain dynamic linking related sections because we linked it minimally with
ld.However, if you compile a C hello world with GCC 8.2:
gcc -o main.out main.csome other interesting sections would appear.
Contains the path to the dynamic loader, i.e.
/lib64/ld-linux-x86-64.so.2 in Ubuntu 18.10. Explained at: stackoverflow.com/questions/8040631/checking-if-a-binary-compiled-with-static/55664341#55664341 Classification of k-transitive groups by Ciro Santilli 35 Updated 2025-03-28 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-03-28 +Created 1970-01-01TODO this would give a better motivation for the Mathieu group
Contains a lot of different flag masks.
Pinned article: ourbigbook/introduction-to-the-ourbigbook-project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
We have two killer features:
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
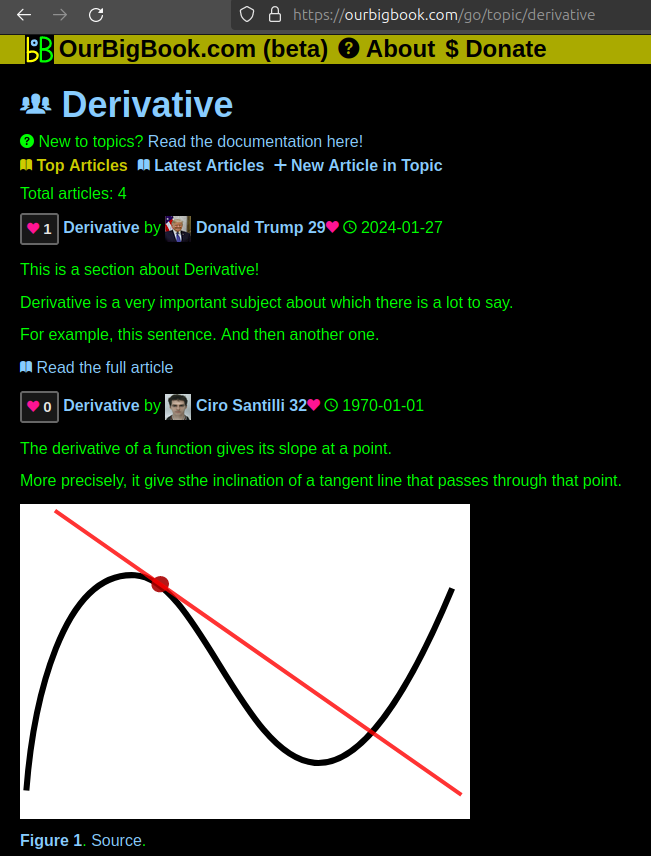
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivative - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
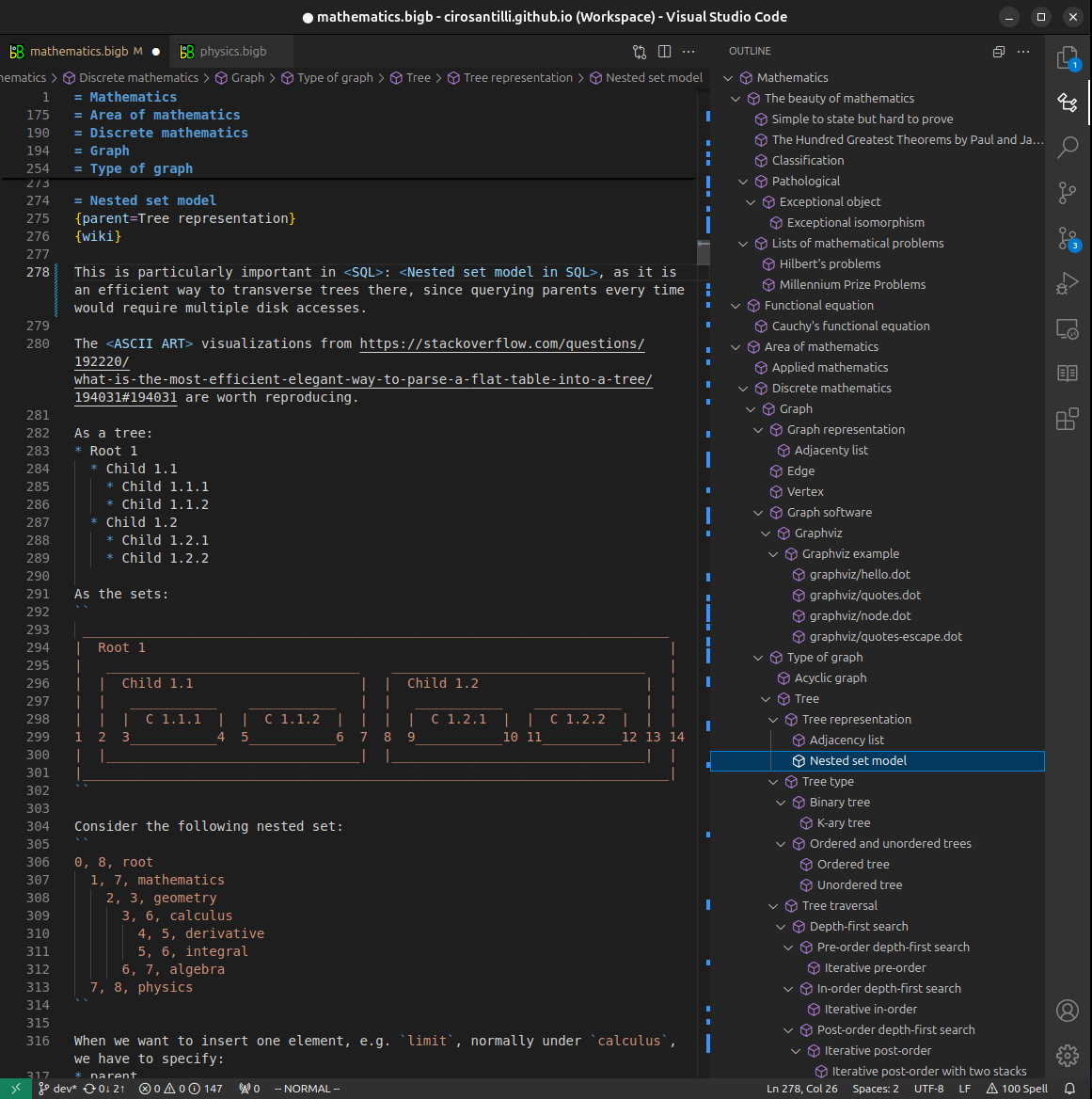
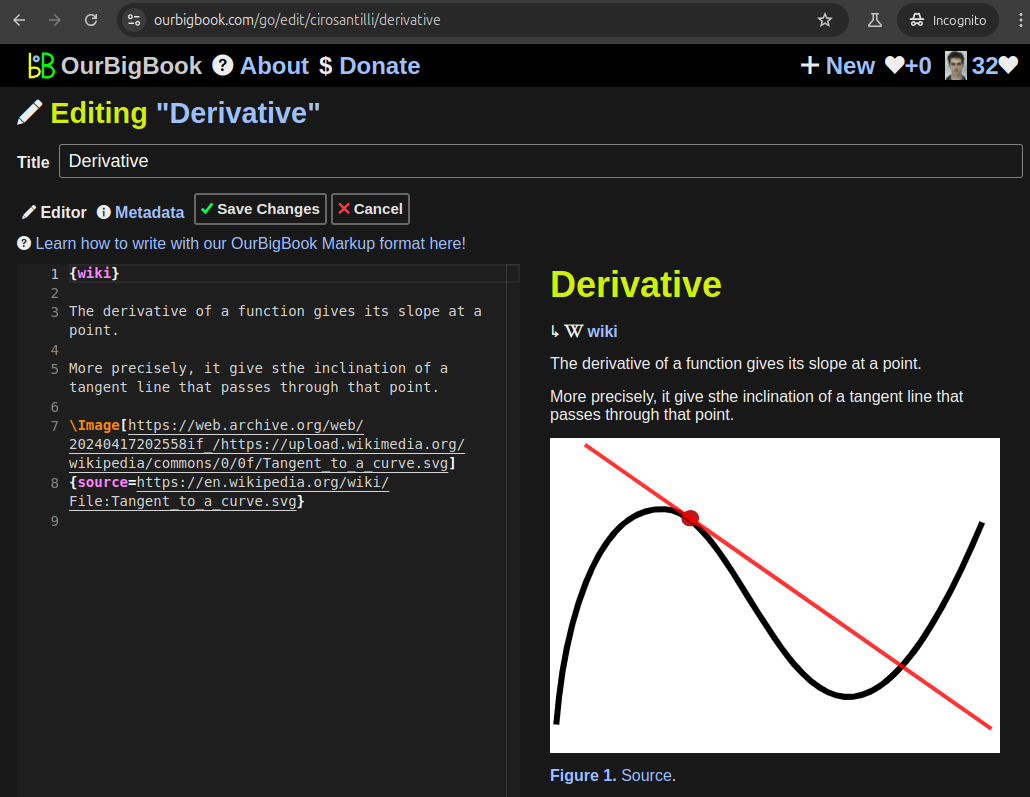
Figure 2. You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website.
Figure 3. Visual Studio Code extension installation.
Figure 4. Visual Studio Code extension tree navigation.
Figure 5. . You can also edit articles on the Web editor without installing anything locally. Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension.Video 4. OurBigBook Visual Studio Code extension editing and navigation demo. Source. - Internal cross file references done right:
- Infinitely deep tables of contents:
Figure 6. Dynamic article tree with infinitely deep table of contents.Live URL: ourbigbook.com/cirosantilli/chordateDescendant pages can also show up as toplevel e.g.: ourbigbook.com/cirosantilli/chordate-subclade
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact