Medieval European mathematics refers to the mathematical developments and practices that occurred in Europe during the Middle Ages, roughly from the 5th to the late 15th century. This period was characterized by various influences, including the transmission of knowledge from ancient civilizations, the rise of universities, and the interactions with Islamic mathematicians and scholars.
A field can be seen as an Abelian group that has two group operations defined on it: addition and multiplication.
And then, besides each of the two operations obeying the group axioms individually, and they are compatible between themselves according to the distributive property.
Examples:
Mathematics in the medieval Islamic world, often referred to as Islamic mathematics, was a significant and transformative field that flourished from the 8th to the 15th centuries. This period was marked by a remarkable exchange of knowledge, as scholars built upon the mathematical foundations of ancient civilizations such as the Greeks, Romans, Indians, and Persians.
Japanese mathematics refers to both historical and contemporary mathematical practices and developments in Japan. The term can encompass a variety of topics, including traditional Japanese mathematics (often called "wasan"), modern mathematical education, and contemporary research and contributions to global mathematics. ### Historical Context: Wasan 1. **Wasan (和算)**: This term specifically refers to traditional Japanese mathematics that developed from the 17th century until the 19th century.
so what's that point of "Open" in the name anymore??
- www.technologyreview.com/2020/02/17/844721/ai-openai-moonshot-elon-musk-sam-altman-greg-brockman-messy-secretive-reality/ "The AI moonshot was founded in the spirit of transparency. This is the inside story of how competitive pressure eroded that idealism."
- archive.ph/wXBtB How OpenAI Sold its Soul for $1 Billion
- www.reddit.com/r/GPT3/comments/n2eo86/is_gpt3_open_source/
Chinese mathematics refers to the mathematical practices, theories, and techniques developed and used in China over thousands of years. It has a rich history that includes significant contributions to various fields of mathematics, such as arithmetic, geometry, algebra, and number theory.
Pinned article: ourbigbook/introduction-to-the-ourbigbook-project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
Intro to OurBigBook
. Source. We have two killer features:
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
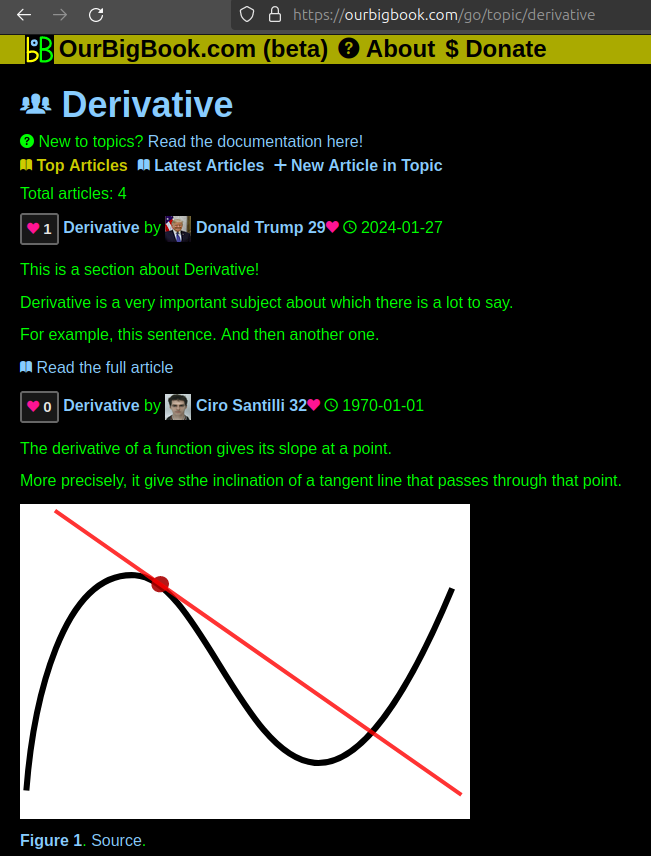
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivativeVideo 2. OurBigBook Web topics demo. Source. - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
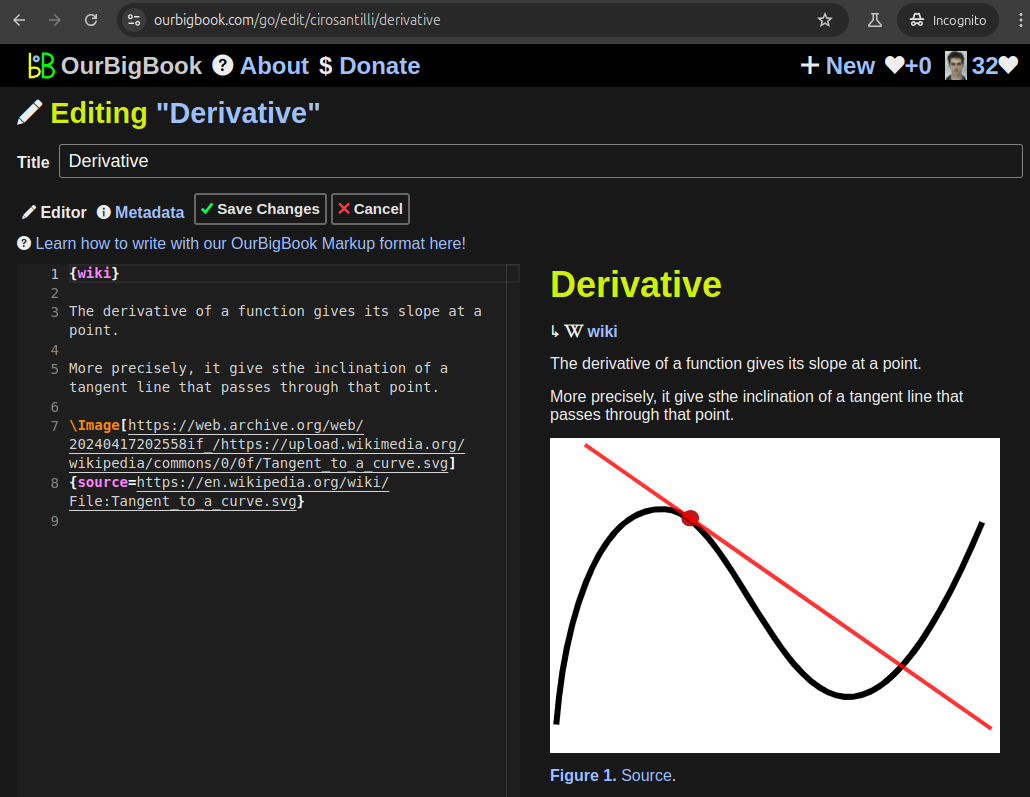
Figure 2. You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website.
Figure 3. Visual Studio Code extension installation.
Figure 5. . You can also edit articles on the Web editor without installing anything locally. Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension. 
- Infinitely deep tables of contents:
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact