Used a lot in quantum mechanics, where the equations are really hard to solve. There's even a dedicated wiki page for it: en.wikipedia.org/wiki/Perturbation_theory_(quantum_mechanics). Notably, Feynman diagrams are a way to represent perturbation calculations in quantum field theory.
Let's gather some of the best results we come across here:
All those dedicated applied mathematicians languages are a waste of society's time, Ciro Santilli sure applied mathematicians are capable of writing a few extra braces in exchange for a sane general purpose language, we should instead just invest in good libraries with fast C bindings for those languages like NumPy where needed, and powerful mainlined integrated development environments.
And when Ciro Santilli see the closed source ones like MATLAB being used, it makes him lose all hope on humanity. Why. As of 2020. Why? In the 1980s, maybe. But in the 2020s?
Techniques to get numerical approximations to numeric mathematical problems.
The entire field comes down to estimating the true values with a known error bound, and creating algorithms that make those error bounds asymptotically smaller.
Not the most beautiful field of pure mathematics, but fundamentally useful since we can't solve almost any useful equation without computers!
The solution visualizations can also provide valuable intuition however.
Important numerical analysis problems include solving:
The "greatest common divisor" of two integers and , denoted is the largest natural number that divides both of the integers.
For example, is 4, because:
- 4 divides both 8 and 12
- and this is not the case for any number larger than 4. E.g.:and so on.
- 5 divides neither one
- 6 divides 12
- 7 divides neither
- 8 divides only 8
Polynomial time for most inputs, but not for some very rare ones. TODO can they be determined?
But it is better in practice than the AKS primality test, which is always polynomial time.
They come up a lot in many contexts, e.g.:
@cirosantilli/_file/prime-number-theorem by 
Ciro Santilli 35 Updated 2025-03-28 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-03-28 +Created 1970-01-01Consider this is a study in failed computational number theory.
The approximation converges really slowly, and we can't easy go far enough to see that the ration converges to 1 with only awk and primes:Runs in 30 minutes tested on Ubuntu 22.10 and P51, producing:
sudo apt intsall bsdgames
cd prime-number-theorem
./main.py 100000000
Linear vs approximation plot
. and are added to give a better sense of scale. is too close to 0 and not visible, and the approximation almost overlaps entirely with .
. It is clear that the difference diverges, albeit very slowly.
. We just don't have enough points to clearly see that it is converging to 1.0, the convergence truly is very slow. The logarithm integral approximation is much much better, but we can't calculate it in awk, sadface.
But looking at: en.wikipedia.org/wiki/File:Prime_number_theorem_ratio_convergence.svg we see that it takes way longer to get closer to 1, even at it is still not super close. Inspecting the code there we see:so OK, it is not something doable on a personal computer just like that.
(* Supplement with larger known PrimePi values that are too large for \
Mathematica to compute *)
LargePiPrime = {{10^13, 346065536839}, {10^14, 3204941750802}, {10^15,
29844570422669}, {10^16, 279238341033925}, {10^17,
2623557157654233}, {10^18, 24739954287740860}, {10^19,
234057667276344607}, {10^20, 2220819602560918840}, {10^21,
21127269486018731928}, {10^22, 201467286689315906290}, {10^23,
1925320391606803968923}, {10^24, 18435599767349200867866}};Let's show them how it's done with primes + awk. Edit. They have a gives us the list of all twin primes up to 100:Tested on Ubuntu 22.10.
-d option which also shows gaps!!! Too strong:sudo apt install bsdgames
primes -d 1 100 | awk '/\(2\)/{print $1 - 2, $1 }'0 2
3 5
5 7
11 13
17 19
29 31
41 43
59 61
71 73There are infinitely many primes with a neighbor not further apart than 70 million. This was the first such finite bound to be proven, and therefore a major breakthrough.
This implies that for at least one value (or more) below 70 million there are infinitely many repetitions, but we don't know which e.g. we could have infinitely many:or infinitely many:or infinitely many:or infinitely many:but we don't know which of those.
The Prime k-tuple conjecture conjectures that it is all of them.
Also, if 70 million could be reduced down to 2, we would have a proof of the Twin prime conjecture, but this method would only work for (k, k + 2).
There are infinitely many prime k-tuples for every admissible tuple.
Generalization of the Twin prime conjecture.
As of 2023, there was no specific admissible tuple for which it had been proven that there infinite of, only bounds of type:But these do not specify which specific tuple, e.g. Yitang Zhang's theorem.
there are infinitely 2-tuple instances with at most a finite bound
Pinned article: ourbigbook/introduction-to-the-ourbigbook-project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
We have two killer features:
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
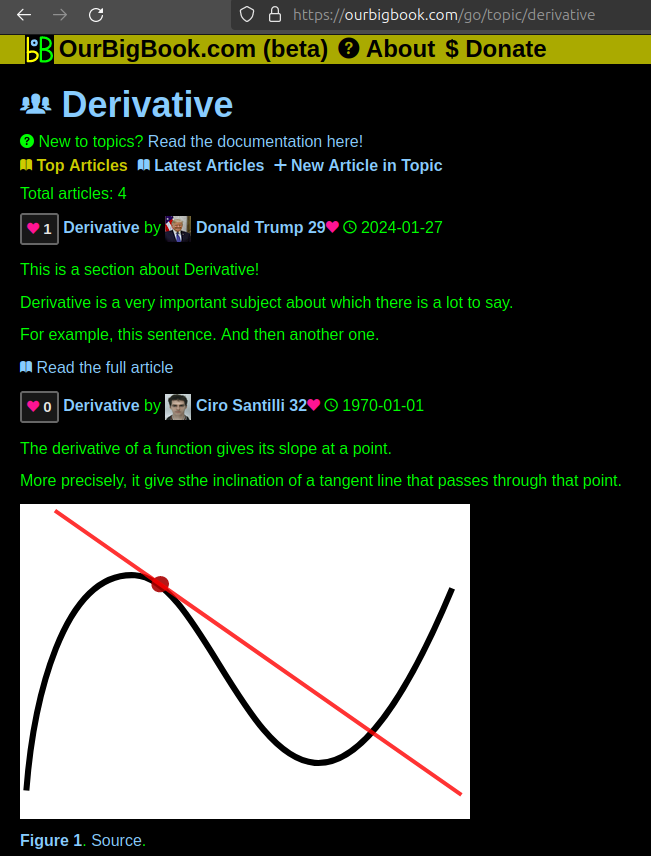
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivative - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
Figure 2. You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website.
Figure 3. Visual Studio Code extension installation.
Figure 4. Visual Studio Code extension tree navigation.
Figure 5. . You can also edit articles on the Web editor without installing anything locally. Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension.Video 4. OurBigBook Visual Studio Code extension editing and navigation demo. Source. - Internal cross file references done right:
- Infinitely deep tables of contents:
Figure 6. Dynamic article tree with infinitely deep table of contents.Live URL: ourbigbook.com/cirosantilli/chordateDescendant pages can also show up as toplevel e.g.: ourbigbook.com/cirosantilli/chordate-subclade
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact