Topics Articles Users Discussions Comments+ New article
New Updated Top Announced A-Z
Advertisement by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Don't like this very much, but if it's the only way...
Maybe focus on job ads like Stack Overflow.
Then:
  • like YouTube, pay creators proportionally to views/metrics
  • paid subscription to remove ads from site
Read the full article
Association with innovative schools by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Maybe we should talk to innovative schools, as they might be more open to such use of technology.
Read the full article
Venture capital by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Not a fun of giving up control for such a low-maintenance cost venture... but keeping a list just in case...
Read the full article
OurBigBook feature by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
Read the full article
OurBigBook topic feature by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Read the full article
OurBigBook dynamic tree by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Read the full article
Ciro Santilli's data projects by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Ciro Santilli has enjoyed doing projects dealing with with lots of data! They usually have a large overlap with Ciro Santilli's naughty projects, but not always!
Read the full article
Ciro Santilli's open source contributions by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
This is mostly stuff from before 2016 when Ciro was anxious to document his contributions to get a job.
Most of the projects here are also minor contributions, or Ciro later noticed that the projects were not useful enough to work on and that he was actually wasting his time.
Read the full article
Patches by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
Read the full article
Merged by others by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Only patches which were reviewed by at least one person with push permission will be listed here.
This may also include patches which were rejected in favor of another patch, but strongly influenced the merged patch.
DateProjectSizeDescription
2024-09python-bitcoin-blockchain-parser1Update plyvel==1.5.1 to fix fatal error: longintrepr.h: No such file or directory build
2024-02btcdeb0fix ‘runtime_error’ is not a member of ‘std’ build error
2019-09KaTeX1Create globalGroup option to place definitions in global scope
2018-06raspi3-tutorial0Explain how to see UART1
2018-05Buildroot1ltp-testsuite: add --with-open-posix-testsuite
2018-03Buildroot1qemu_x86_64_defconfig: fix kernel header version after bump to 4.15
2017-11Cocos2d1Recreate libfmod.so.6 symlinks
2017-10Cocos2d1Make bullet use -fPIC
2017-07OPenCL Headers0C11 anonymous structs / unions should allow xyz notation for vector types
2017-06Linux insides0Mention Buildroot in addition to ivandaviov/minimal
2017-06opengl-tutorial.org0Use existing empty layout on feed.xml
2017-04Tiny Renderer0Add gitignore
2017-02Vulkan Loader0Use nproc instead of ncpu
2017-01Boost Geometry1What is "QPoint::double" in doc/example_adapting_a_legacy_geometry_object_model.qbk ?
2016-12Freetype GL1GLFW Ubuntu package works on 16.10
2016-11honza/vim-snippets1Add C variable printf debug snippets
2016-09Gazebo1Add a world to the gui_overlay_plugin_time example
2016-07Android Vulkan Tutorials1Use android API 24
2016-07minimal linux live0Fix time sh instead of sh time
2016-06GHDL0Typo severals -> several in Invoking doc
2016-06GHDL1Document GCC backend needed to generate executables
2016-06GHDL0Typo debugger -> debugged in BUILD.txt
2016-06GHDL0Typo carray -> carry in full adder example
2016-05facedetect1Add example to extract faces with imagemagick
2016-05ebookFoundation/free-programming-books0Create data science section and add Kaggle to it
2016-05opengl-tutorial.org0Add empty line before triple quotes
2016-05Bullet Physics1Improve HelloWorld
2016-04Freetype GL1Find out how to compile on Ubuntu and document it
2016-03ranger0gitignore install_log.txt generated by make install
2016-03ranger1Add %confdir macro
2016-03ranger1set_bookmark for directories other than the current
2016-02Jami2Create working Android video hardware decoding prototype
2016-02Jami1Started the one command build which was later made official
2016-01leetcode1Java LRUCache with LinkedHashMap
2015-12ebookFoundation/free-programming-books0Add InterviewBit to problem sets
2015-10Linux insides0Recommend ivandaviov/minimal to generate initrd
2015-08bare-metal-tetris0make clean also removes tetris.iso
2015-08eduOS0README typos Binutils and NASM
2015-08GDB0python: fix Linetable case to LineTable in docstrings and comments
2015-07GDB0Remove outdated comment from call-rt-st.exp
2015-07GDB0Fix broken CONTRIBUTE link to GNU insignificant changes
2015-07GDB1py-linetable.c: Fix doc of LineTable.source_lines' return type
2015-07Binutils1Clarify case requirements for gas pseudo-ops
2015-07Capstone0Website typos at beyond_llvm
2015-07Vim Session1auto_save_to option added
2015-05NASM0doc: typo occationally
2015-05ebookFoundation/free-programming-books0Computer Science from the Bottom Up
2015-05ebookFoundation/free-programming-books0problem-sets: leetcode
2015-05ebookFoundation/free-programming-books0Papers we love
2015-04alternativeTo0icanprove.com
2015-04ebookFoundation/free-programming-books0Knapsack Problems
2015-04ebookFoundation/free-programming-books0LSB
2015-04honza/vim-snippets0java: prinlna to print array
2015-04ebookFoundation/free-programming-books0PEG Judge
2015-03GitLab1Convert many JavaScript # links to buttons
2015-03GitLab1Factor permission check in issuable finder
2015-03Spring0Typo Gardle -> Gradle
2015-02Linux insides0Make x86 paging reference title clearer
2015-02GitLab1Ignore .bundle
2015-02ebookFoundation/free-programming-books0Problem sets: CareerCup
2015-01honza/vim-snippets1Fix false positive hyphen list item expansion
2015-01GitLab1Append in place for strings and arrays
2015-01GitLab1Remove unneeded password_confirmation from seed
2015-01GitLab0Typo in project API events comment
2015-01GitLab1Replace match via get with get on routes
2015-01GitLab0Remove or prepend underscore _ to unused method arguments
2015-01GitLab1Change always passing visible false tests
2015-01GitLab1Make blob new and edit file editors more uniform
2015-01GitLab1Replace regex methods by string ones
2015-01GitLab1Simplify SSH fingerprint regexp extraction
2015-01GitLab1Add tests for disabled blob edit button cases v2
2014-12GitLab0permission.md align table, rm double empty line
2014-12GitLab0Remove commit indicator from path on Commits tab
2014-12GitLab0Make protected branch perms explicit in doc
2014-12GitLab0doc workflow markdown style
2014-12GitLab1Disallow POST to compare as it does not create objects
2014-12GitLab0Sort .gitignore
2014-12GitLab1Remove unused Projectcode function
2014-12GitLab0Remove unused has_gitlab_shell3? method
2014-12GitLab1Add tests for tree edit routes
2014-12GitLab1Disable / hide MR edit blob button if cannot edit
2014-12GitLab0Use blob local instead of instance
2014-12GitLab1Fix dev user seed: ID was used twice
2014-12GitLab0Fix Rake tasks doc README: add top level h1
2014-12Ruby0Typo close -> closes.
2014-12CommonMark1Add tests for normalize outer whitespace removal
2014-12CommonMark1spec_tests make --pattern affect --dump-tests
2014-12CommonMark1Add --number option to run a single test by its id
2014-12CommonMark1Don't raise exception on invalid UTF-8 output
2014-12CommonMark1spec_tests add short options for common parameters
2014-12CommonMark0Ignore pyc files
2014-12CommonMark1Expose failure to normalize whitespaces
2014-11honza/vim-snippets1Markdown bold and italic
2014-11honza/vim-snippets1Markdown links URLs from the clipboard
2014-11honza/vim-snippets1Markdown autolinks
2014-11honza/vim-snippets1Let the short version of links not have title.
2014-11honza/vim-snippets1Fix markdown fenced code blocks.
2014-11GitLab1Delete tags and branches that start with hyphen
2014-11GitLab3Restore hooks PATH before calling ruby
2014-11GitLab1Factor regex error messages with spec API tests
2014-11GitLab1Move new blob commit message textarea below editor
2014-11gitbrute0Usage
2014-11GitLab1Factor GITLAB_SHELL_VERSION get method
2014-11GitLab1Create dev fixture projects with fixed visibility
2014-11GitLab1Factor using Repositorypath_to_repo
2014-11GitLab1Remove unused authenticate_user from projectshow
2014-11GitLab1Remove dead Eventnew_branch? method
2014-11GitLab1Don't output to stdout from lib non-interactive methods
2014-11GitLab2Fix version of test seed branches to specific revisions
2014-11GitLab1Factor '0' * 40 blank ref constants
2014-11GitLab1Transform remove blob link into button
2014-11GitLab1Update default regex message to match regex
2014-11GitLab0Continue strings with backslash instead of append
2014-11GitLab1Factor behaviors.scss constants
2014-11GitLab0Remove unneeded backslash: "/" == "/"
2014-11GitLab1Fix push not allowed to protected branch if commit starts with 7 zeros
2014-11GitLab1Use require spec_helper instead of relative path
2014-11GitLab0Fix doc rake import md style
2014-11GitLab1Factor lib backend gitlab shell path
2014-10GitLab1Run user select Js only where needed
2014-10GitLab1Use button type=submit instead of input
2014-10GitLab1Only run profile js on pages that need it
2014-10GitLab1Better js -> URL projects map to reduce unneeded execution
2014-10GitLab1Use Gitlab.config instead of Settings everywhere
2014-10GitLab1Show nothing instead of unassigned on issues
2014-10GitLab1Only run namespace select js when needed
2014-10GitLab0Merge File basename and dirname into split
2014-10GitLab1Fix import.rake failed import if project name is also an existing namespace
2014-10GitLab0Remove unused variable user at lib/gitlab/markdown
2014-10GitLab1Use argument list for sh instead of string
2014-10GitLab1Only run avatar chooser Js on pages that need it
2014-10GitLab1Remove whitespace link between user group avatars
2014-10GitLab0Fix doc raketasts import md style
2014-10GitLab1Remove unneeded app/finders config.autoload path
2014-10GitLab0Improve grack auth hooks comment.
2014-10GitLab1Remove unused admin/projectsrepository method
2014-10GitLab1Factor admin logs
2014-10GitLab1Remove unused filter from ProjectsController
2014-10GitLab1Remove unused dev_tools helper.
2014-10GitLab1Factor authorize_push! and authorize_code_access!
2014-10GitLab1Replace match with end_with: more readable, faster
2014-10GitLab1Use @project on controllers, don't call method
2014-10GitLab1Remove param[:project_id] at admin controller
2014-10GitLab1DRY mentioned in magic note constant
2014-10GitLab1Factor group forms
2014-10GitLab1State on CONTRIBUTING that people should fix line style of touched lines
2014-10GitLab1Export all coffee classes with @
2014-10GitLab1Fix missing flash on file edit error from web UI.
2014-10Capybara0Fix History typo.
2014-10GitLab1Make new and edit file submit more uniform
2014-10libgit21Join typedef and struct definitions in single file
2014-10GitLab1Factor dashboard helper methods
2014-10GitLab1Factor issue and merge request services
2014-10GitLab1Replace www.gitlab.com with about.gitlab.com
2014-10GitLab0Improve formatting of app/finders/README.md
2014-10GitLab0Remove outdated comment from commits_controller
2014-10GitLab1Factor markup? gitlab_markdown? into new method
2014-10GitLab1Remove unused title parameter
2014-10GitLab1Make Spinach test names consistent
2014-10GitLab0Ignore .bundle
2014-10GitLab0Ignore tags file
2014-10GitLab0Split one instance variable per line
2014-10GitLab1Factor commit message textareas
2014-10GitLab1Remove outdated comment on the project test seed
2014-10GitLab0Remove assignment without effect.
2014-10GitLab1Add parenthesis to function def with arguments.
2014-10GitLab1Remove test line without effect because no should.
2014-10GitLab1Improve remove file commit message textarea placeholder
2014-10GitLab1Replace :erb filter with plain HAML
2014-10GitLab1Remove blame lines added leading whitespace
2014-10GitLab1Improve new file commit message textarea placeholder.
2014-10GitLab1Simplify custom MR good commit message hint
2014-10GitLab1Move group feature step to match test location
2014-10GitLab1Titleize blob action buttons.
2014-09GitLab0Remove statement without effect.
2014-09GitLab0Fix dev merge seed: update testme to gitlab-test.
2014-09GitLab0Remove trailing whitespace from views.
2014-09GitLab1Remove def project from tests that inherit it.
2014-09GitLab1Replace testme with gitlab-test.
2014-09GitLab1Add predictable merge requests on dev seed.
2014-09GitLab1Prevent email sending on admin dev seed.
2014-09GitLab1Only show text wrap and diff notes for text in merge requests.
2014-09GitLab1Add web UI file CRUD tests.
2014-09GitLab1Remove type submit from button_tag since default.
2014-09GitLab1Replace empty? nil? with blank?.
2014-09GitLab0Typo indiciated -> indicated.
2014-09GitLab1Remove unnecessary page. from tests.
2014-09GitLab1Remove ununsed CONTRIBUTING link on edit MR form.
2014-09GitLab1Add g++ dependency to ubuntu install.
2014-09GitLab0Hound prefer single quotes.
2014-09libgit20Remove unused buf variable from path/core test.
2014-09GitLab1Only clone GitLab Shell on tests if necessary.
2014-09GitLab1Factor fork button view.
2014-09GitLab1evaluate -> execute_script when return not needed.
2014-09GitLab1evaluate_script history -> go_back and go_forward.
2014-09GitLab1Factor current_url + URI.path into current_path.
2014-09GitLab1Replace javascript:; links with buttons.
2014-09GitLab1Factor .add-diff-note active state.
2014-09GitLab1Fix link_to_reply_diff.
2014-09GitLab1Factor issue and MR edit form error list.
2014-09GitLab1Factor error and success methods from services.
2014-09GitLab1Set textarea resize:vertical by default.
2014-09GitLab1Factor out commit list from compare and new MR.
2014-09GitLab1Prefix Spinach features with Spinach::Features::.
2014-09GitLab0Typo it -> its.
2014-09GitLab1Factor zen mode.
2014-09GitLab0Ignore tags file.
2014-09GitLab1Improve zen mode internals.
2014-09GitLab0CONTRIBUTING typos.
2014-09Marked1Add browser usage to README
2014-09GitLab0Typo herlper -> helper.
2014-09libgit21Factor 40 and 41 constants from source.
2014-09libgit21Replace void casts with GIT_UNUSED.
2014-09libgit2/rugged0Typo "di ff" -> diff.
2014-09libgit2/rugged0Remove trailing whitespace.
2014-09libgit2/rugged0Gitignore rdoc/.
2014-09libgit2/rugged0Factor File.join in test sandbox_init.
2014-09Ruby on Rails1Explain ERB space removal.
2014-09GitLab0Update README to match Md style in CONTRIBUTING.
2014-09GitLab0Typo localy -> locally
2014-09vader.vim1Add run-tests script.
2014-09vader.vim2Add SyntaxAt and SyntaxOf helpers.
2014-09libgit2/rugged0Remove trailing whitespace.
2014-09libgit2/rugged0Gitignore rdoc/.
2014-09libgit2/rugged0Factor File.join in test sandbox_init.
2014-09Pro Git book1Mention packed-refs.
2014-09GitLab1Add link to fixed SHA version on blob.
2014-09GitLab1Factor new issue and edit MR forms.
2014-09GitLab1Fix missing to on reassign Merge Request text email to unassigned.
2014-09GitLab1Fix missing to on reassign Merge Request email to unassigned.
2014-09karlcow/markdown-testsuite1Run multimarkdown in compatibility mode.
2014-09karlcow/markdown-testsuite1Link to stmd.
2014-09GitLab1Add users with deterministic username and password to development seed.
2014-09Ruby on Rails0Shorten ActionView::Base doc summary line.
2014-09Ruby on Rails1Clarify Rails uses erubis not stdlin ERB.
2014-08honza/vim-snippets1Rename node p to pipe
2014-08GitLab0Typo.
2014-08honza/vim-snippets1README improvements: md style, typos, fix links.
2014-08honza/vim-snippets1Add tex hyperlink snippets.
2014-08GitLab1Remove HAML eval for const strings.
2014-08softcover1Ignore template top level tex file.
2014-08softcover1Fix failing PDF fontsize verbatim test.
2014-08honza/vim-snippets1Add tex listings snippets.
2014-08GitLab0Fix Md style for API docs.
2014-08GitLab0Fix Md style for projects API doc.
2014-08GitLab1Restrict commit area resize to vertical.
2014-08GitLab0Update README Markdown style to match CONTRIBUTING
2014-07Pro Git book1Set dummy merge driver merge ours .gitattributes.
2014-07GitLab2Add project stars.
2014-07GitLab1Increase diff byte highlight contrast.
2014-07GitLab0Clarify squash comes after review.
2014-07GitLab0Enforce Markdown style.
2014-07Octokat.js1Gitignore fixtures and dist/commonjs.
2014-07GitLab1Fix username validation message to match regexp.
2014-06GitLab1Add trailing newline to all text files.
2014-06GitLab0Typo.
2014-06markdownlint/markdownlint0Typo.
2014-06Ruby on Rails0Typo.
2014-06GitLab1Replace HTML5 obsolete center element with CSS.
2014-06developer.github.com1Explain :user is username not ID.
2014-06Prose0Correct CONTRIBUTING typos.
2014-06Octokat.js1Fix repo to repos in README examples.
2014-06Octokat.js1Fix typos and style on README.
2014-06octokit.js0Remove trailing whitespace.
2014-06octokit.js1Add grunt watch.
2014-06Octokat.js0Remove unneeded semicolon.
2014-06Octokat.js1Add grunt watch.
2014-06karlcow/markdown-testsuite2Add Vagrantfile.
2014-06karlcow/markdown-testsuite1Remove hoedown options.
2014-06honza/vim-snippets1Add HTML ac Anchor from Clipboard.
2014-06RVM0Correct some doc typos.
2014-06GitLab1Clarify that bbastov is the style of Hound CI.
2014-06GitLab2Update docs to match new markdown style guide.
2014-05karlcow/markdown-testsuite1Add blackfriday, lunamark, maruku and rdiscount.
2014-05karlcow/markdown-testsuite1Add autolink-no-bracket extension test.
2014-05karlcow/markdown-testsuite0Add showdown engine.
2014-05karlcow/markdown-testsuite1Title attribute is significant at normalization.
2014-05karlcow/markdown-testsuite1Add design goals.
2014-05karlcow/markdown-testsuite0Add Python Markdown 2 engine.
2014-05karlcow/markdown-testsuite0Add peg-markdown engine.
2014-05GitLab1Commit message textareas have 72 char mark line.
2014-05karlcow/markdown-testsuite1Add autolink-no-bracket extension test.
2014-05karlcow/markdown-testsuite1Add link-idref-implicit-no-bracket test.
2014-05karlcow/markdown-testsuite1Minor fixes to addition of hoedown.
2014-05karlcow/markdown-testsuite1Remove no-auto-id argument for kramdown.
2014-05karlcow/markdown-testsuite1Add markdown_pl Markdown.pl engine.
2014-04GitLab0Remove redundant signin link from signin page.
2014-04GitLab1Add help link to header.
2014-04karlcow/markdown-testsuite1Improve output normalization with custom parser.
2014-04karlcow/markdown-testsuite1Add ordered-list-inner-par-list test.
2014-04GitLab CI1Add application.yml.example.development.
2014-04Boost Graph1Explicitly use vertex type on quick tour example.
2014-04karlcow/markdown-testsuite1Add list-code-1-space test.
2014-04karlcow/markdown-testsuite1Add md2html engine.
2014-04karlcow/markdown-testsuite1Remove email tests because output is random.
2014-04karlcow/markdown-testsuite2Run only tests that contain string in title.
2014-04karlcow/markdown-testsuite1Add marked engine.
2014-04GitLab1Add markdown styleguide.
2014-04GitLab1Include SASS in subdirectories with glob.
2014-04tig1Add refs bind ! to delete branch.
2014-04GitLab1Rename issue form tags to match MR and params.
2014-04GitLab1Say issues are accepted at both GitLab and GitHub.
2014-03karlcow/markdown-testsuite1Document config_local.py on README.
2014-03karlcow/markdown-testsuite1Factor out engines that are commands on PATH.
2014-03karlcow/markdown-testsuite1Add sample run-tests.py output to README.
2014-03karlcow/markdown-testsuite1Check if are no engines enabled to avoid exception.
2014-03plasticboy/vim-markdown1Add Toc commands.
2014-03karlcow/markdown-testsuite0One disable per line commented out on conf.
2014-03karlcow/markdown-testsuite0Add multimarkdown support.
2014-03karlcow/markdown-testsuite0Typo conten -> content.
2014-03karlcow/markdown-testsuite2Automated tests.
2014-03GitLab CI0Remove config/puma.rb from gitignore.
2014-03GitLab CI0Ignore config/unicorn.rb.
2014-03GitLab CI0Tell users to install bundle locally without sudo.
2014-03GitLab CI0Document where to find the registration token.
2014-03karlcow/markdown-testsuite0Remove space from simple list, specify asterisk.
2014-03karlcow/markdown-testsuite1Add script to cat all input files.
2014-03karlcow/markdown-testsuite0Remove newline from empty files.
2014-03GitLab1Start development Key seed id from 1.
2014-03GitLab1Show link to public projects for new users.
2014-03GitLab cookbook1Correct bindfs metal dev init script.
2014-03GitLab CI0Organize gitignore.
2014-03GitLab cookbook1Correct metal install home share technique.
2014-03GitLab cookbook0Uniform markdown headers
2014-03GitLab cookbook0Typo ommited -> omitted.
2014-03GitLab0Documentation Typos
2014-02plasticboy/vim-markdown1Add contributing guidelines and started tests as required by them.
2014-02plasticboy/vim-markdown0Add Vundle install to README, updated pathogen URL to GitHub.
2014-02plasticboy/vim-markdown0Create credits section, remove link to old homepage.
2014-02GitLab1Remove dir prefix from filename of tests under dir.
2014-02karlcow/markdown-testsuite0Make title more readable.
2014-02karlcow/markdown-testsuite0Make readme intro more direct.
2014-02karlcow/markdown-testsuite1Add extensions.
2014-02GitLab2Blob and tree markdown links to anchors work.
2014-02git-browse-remote0Add install instructions.
2014-02Overleaf1Remove latexmk install instructions from README.
2014-02Overleaf1Remove dollars from readme bash code.
2014-02Overleaf1Add dummy version to package.json to fix install.
2014-02Overleaf1Add .nvmrc
2014-02GitLab2User can leave group from group page.
2014-02GitLab2Add anchors to markdown rendered headers.
2014-02GitLab2User profile pages are publicly visible.
2014-01GitLab cookbook1Development install documentation correction.
2014-01GitLab cookbook2Create metal development install documentation.
2014-01GitLab cookbook0Improve docs.
2014-01GitLab cookbook1Add option to control the SSH port used.
2013-12GitLab cookbook1Improve production install docs.
2013-11plasticboy/vim-markdown1Header navigation mappings work for Setext headers.
2013-10okular1Add shortcut to Change Colors on a page.
2013-09plasticboy/vim-markdown2Add mappings to navigate across headers.
2013-02SciPy1Improve documentation.
2013-01Django Userena1Add new configuration option.
2012-11DataTables1Improved a doc example.
Read the full article
Merged by Ciro by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Patches which were merged by Ciro himself on repositories which eh feels have large public visibility, e.g. those to which he has been given push permission.
Repositories to which Ciro gained push permission because of his contributions:
Read the full article
Open source by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
DateProjectSizeDescription
2019-04gnuplotWhy does plotting with point labels make plot generation extremely slow?
2019-04GDB DashboardLimit the size of shown arguments in the Stack display
2018-03QEMU2Test record and replay feature. Also here
2018-02pandocAdd option to produce AsciiDoc output without explicit heading ids
2017-10AndroidGLES3 content gles3jni from ndk examples fails with "java.lang.RuntimeException: createContext failed: EGL_BAD_CONFIG"
2017-09Mozilla rrHow to automatically start replay and go directly to main instead of _start?
2017-09Mozilla rrReverse step over time(NULL) enters rr/src/preload/syscall_hook.S and leads to "Cannot find bounds of current function"
2017-08xselWhy maximum 4000 characters output with xsel -b ?
2017-06BuildrootDon't print mutiline struct function arguments on stack when set pretty print on
2017-04GDB DashboardAdd style option to print stack arguments on a single line
2017-05BuildrootBuild fails with "unexpected EOF while looking for matching "'" if PATH contains a newline
2017-04GDB DashboardAdd style option to print stack arguments on a single line
2017-03clBLAS.s[0] + CL_DEVICE_TYPE_ALL
2017-01game-icons.netUse multiple separate paths, allow customizing the color of each component, and give a default color
2017-01game-icons.netdelapouite/originals/svg/brick-wall.svg has some whitespace on top
2017-01OpenAI Gymexamples/agents/keyboard_agent.py fails with "AttributeError: 'TimeLimit' object has no attribute 'viewer'"
2016-12Simple DirectMedia LayerAdd C variable printf debug snippets
2015-03tigAccepted feature.
2014-11GitLabDuplicate
2014-11GitLabBug.
2014-11GitLabSupport.
2014-11Bootstrap Hover DropdownBug confirmed.
2014-11GitLabBug confirmed.
2014-11GitLabTriaging.
2014-11GitLabProblem with the display icons in the left block
2014-11sassBug confirmed.
2014-10GitLabPoint duplicate.
2014-10GitLabBug confirmed.
2014-10GitLabBug confirmed.
2014-10Semaphore CIBug confirmed.
2014-10libgit2Bug confirmed.
2014-10GitLabSupport.
2014-10GitLabPoint duplicate.
2014-09vader.vimAccepted feature.
2014-09GitLabPoint already fixed.
2014-09vader.vimAccepted feature.
2014-09GitLabBug confirmed.
2014-09GitLabBug confirmed.
2014-09GitLabPoint duplicate.
2014-09GitLabPoint already fixed.
2014-08markdownlint/markdownlintAccepted feature.
2014-08softcoverAccepted feature.
2014-08markdownlint/markdownlintAccepted feature.
2014-07GitLabBug confirmed.
2014-07GitLabAccepted feature.
2014-07GitLabAccepted feature.
2014-06GitLabAccepted feature.
2014-06GitLabPoint duplicate.
2014-06karlcow/markdown-testsuiteBug confirmed.
2014-06plasticboy/vim-markdownClose issue.
2014-06plasticboy/vim-markdownReview patch.
2014-06plasticboy/vim-markdownReview and patch patch.
2014-05softcoverAccepted feature.
2014-04karlcow/markdown-testsuiteClose issue with better issues.
2014-03tigAccepted feature.
2014-03GitLabAccepted feature.
2014-03softcoverAccepted feature.
2014-03GitLabAdd useful information.
2014-03GitLabPoint duplicate.
2014-03GitLabPoint duplicate.
2014-03GitLabAccepted feature.
2014-02GitLabPoint duplicate.
2014-02GitLabAccepted feature.
2014-02OverleafFeature generated considerable interest.
2014-02GitLabPoint already fixed.
2014-02GitLabLink feature request to patch.
2013-10yakuakeBug confirmed.
2013-10okularBug confirmed.
2013-06krusaderBug confirmed.
2013-05NumPyBug confirmed + inner cause.
2012-05krusaderAccepted feature.
2012-05krusaderBug confirmed.
2012-05AutoKeyBug confirmed.
Read the full article
Not verified by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
The following contributions where not immediately verified by others, but they were not reverted either and I think they are good.
DateProjectTypeDescription
2014-12plasticboy/vim-markdownReview patchAdd tilde-fenced code blocks
Read the full article
Twin prime by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
Read the full article
Mathieu group by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Contains the first sporadic groups discovered by far: 11 and 12 in 1861, and 22, 23 and 24 in 1973. And therefore presumably the simplest! The next sporadic ones discovered were the Janko groups, only in 1965!
Each is a permutation group on elements. There isn't an obvious algorithmic relationship between and the actual group.
TODO initial motivation? Why did Mathieu care about k-transitive groups?
Their; k-transitive group properties seem to be the main characterization, according to Wikipedia:
  • 22 is 3-transitive but not 4-transitive.
  • four of them (11, 12, 23 and 24) are the only sporadic 4-transitive groups as per the classification of 4-transitive groups (no known simpler proof as of 2021), which sounds like a reasonable characterization. Note that 12 and 25 are also 5 transitive.
Looking at the classification of k-transitive groups we see that the Mathieu groups are the only families of 4 and 5 transitive groups other than symmetric groups and alternating groups. 3-transitive is not as nice, so let's just say it is the stabilizer of and be done with it.
Video 1.
Mathieu group section of Why Do Sporadic Groups Exist? by Another Roof (2023)
Source. Only discusses Mathieu group but is very good at that.
Read the full article
Selected screenshots by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Figure 1.
2010 Wayback Machine archive of starwarsweb.net
.
The Star Wars one. Clearly branded websites like this are rare, which makes finding them all the much more fun. The Reuters article had two of them (Carson and rastadirect.net), so these were probably manually selected from the full hit dataset, and did not serve specifically as entry points. Most of the websites are quite boring and forgetful as you'd expect.
The subtitle "Beyond The Unknown" may be a reference to the Unknown Regions in the Star Wars fictional universe.
Figure 2.
2011 Wayback Machine archive of iranfootballsource.com
. The third Iranian football on top of the two other published by Reuters: iraniangoalkicks.com and iraniangoals.com! Admittedly, this one is the most generic and less well designed one. But still. They pushed the theme too far!
Figure 3.
2010 Wayback Machine archive of dedrickonline.com
.
The German one.
The CIA has had a few Germany espionage scandals in the 2010s:
Figure 4.
2010 Wayback Machine archive of lesummumdelafinance.com
. A French one. Because it mentions VTT (Mountain Biking in French), it must focus France.
Figure 5.
2011 Wayback Machine archive of attivitaestremi.com
. An Italian one about extreme sports.
Figure 6.
2010 Wayback Machine archive of noticiasmusica.net
.
The Brazilian one.
Figure 7.
2011 Wayback Machine archive of economicnewsbuzz.com
. The Korean one. Love the kawaii style!
Figure 8.
2011 Wayback Machine archive of snapnewsfront.net
. The Japanese one.
Figure 9.
2010 Wayback Machine archive of philippinenewsonline.net
. The Philippine one one.
Figure 10.
2011 Wayback Machine archive of feedsdemexicoyelmundo.com
. The Mexican one.
Figure 11.
2012 Wayback Machine archive of easytraveleurope.com
.
Figure 12.
2011 Wayback Machine archive of tee-shot.net
. One of the many golf-themed sites. Golf appears to be quite popular over in Langley. It's exactly what you'd expect for a mid-level spook to do in their free time!
Figure 13.
2011 Wayback Machine archive of nouvellesetdesrapports.com
.
Figure 14.
2011 Wayback Machine archive of pangawana.com
.
Figure 15.
2011 Wayback Machine archive of recuerdosdeviajeonline.com
.
Figure 16.
2011 Wayback Machine archive of theworld-news.net
.
Figure 17.
2011 Wayback Machine archive of kessingerssportsnews.com
.
Figure 18.
2011 Wayback Machine archive of negativeaperture.com
.
Read the full article
Backlinks by Ciro Santilli 35 Updated 2025-02-26 +Created 1970-01-01
View more
Initial announcements by self on 2023-06-10:
Shared by others soo after:
2023-10-26 twitter.com/cirosantilli/status/1717445686214504830: announcement by self after finding 75 more sites
Second wave:
Some more:
/ny
Read the full article

Pinned article: ourbigbook/introduction-to-the-ourbigbook-project

Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
Video 1.
Intro to OurBigBook
. Source.
We have two killer features:
  1. topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculus
    Articles of different users are sorted by upvote within each article page. This feature is a bit like:
    • a Wikipedia where each user can have their own version of each article
    • a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
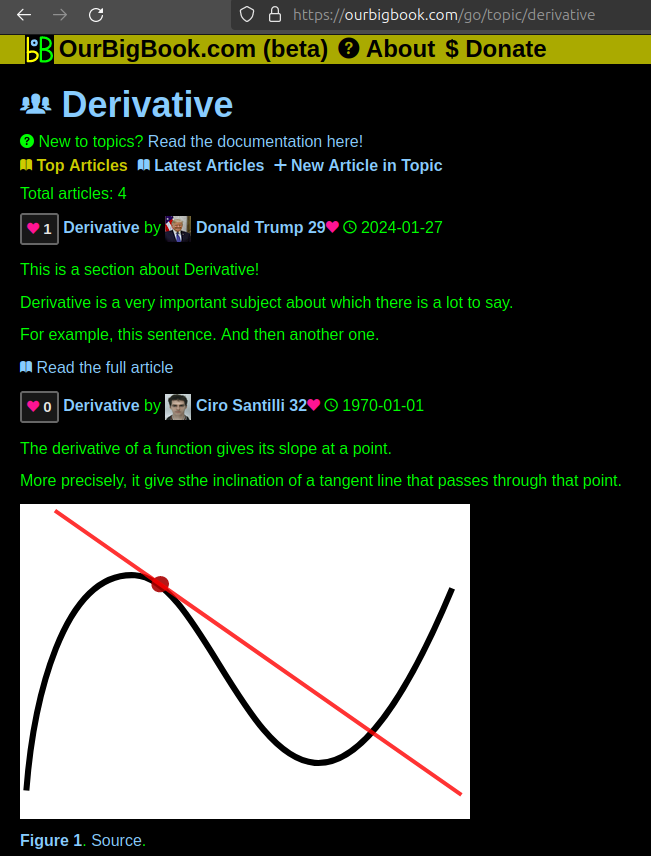
    This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.
    Figure 1.
    Screenshot of the "Derivative" topic page
    . View it live at: ourbigbook.com/go/topic/derivative
    Video 2.
    OurBigBook Web topics demo
    . Source.
  2. local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:
    • to OurBigBook.com to get awesome multi-user features like topics and likes
    • as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
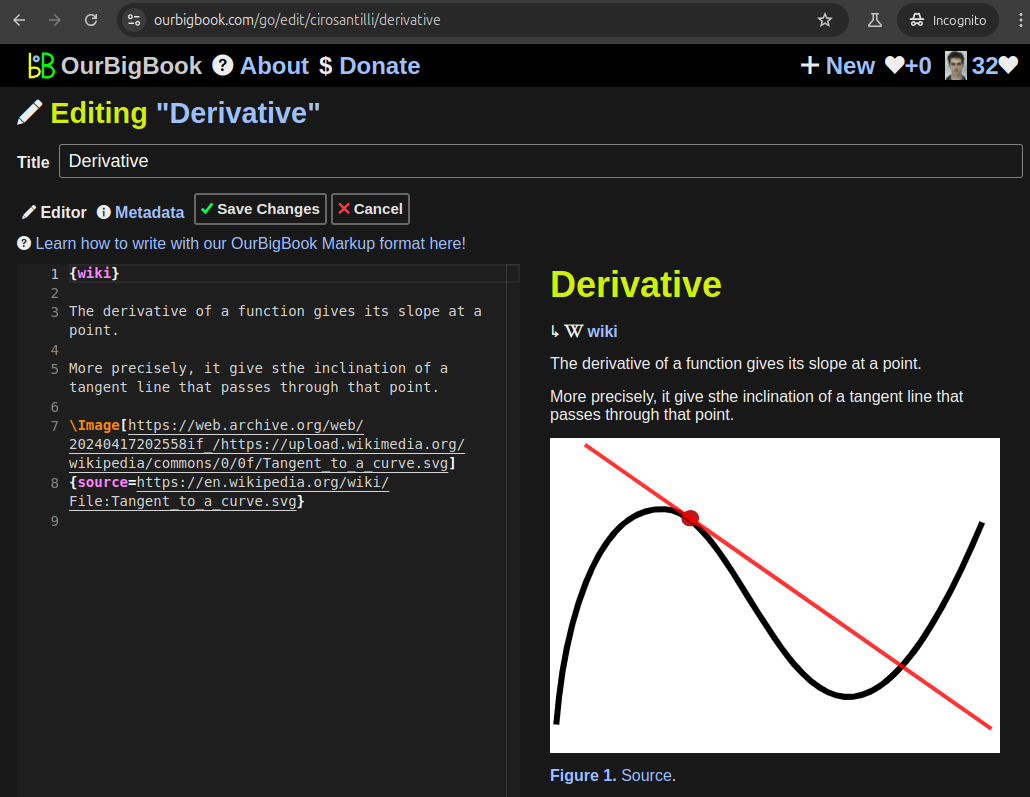
    This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
    Figure 2.
    You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website
    .
    Figure 3.
    Visual Studio Code extension installation
    .
    Figure 4.
    Visual Studio Code extension tree navigation
    .
    Figure 5.
    Web editor
    . You can also edit articles on the Web editor without installing anything locally.
    Video 3.
    Edit locally and publish demo
    . Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension.
    Video 4.
    OurBigBook Visual Studio Code extension editing and navigation demo
    . Source.
  3. https://raw.githubusercontent.com/ourbigbook/ourbigbook-media/master/feature/x/hilbert-space-arrow.png
  4. Infinitely deep tables of contents:
    Figure 6.
    Dynamic article tree with infinitely deep table of contents
    .
    Descendant pages can also show up as toplevel e.g.: ourbigbook.com/cirosantilli/chordate-subclade
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact
Site settings