www.unilad.com/technology/erik-finman-bitcoin-12-year-old-millionaire-invest-798094-20231207
Shame that he seems to be a American exceptionalism idiot. Perhaps it was inevitable given his circonstances. After a small market crash: x.com/erikfinman/status/1820457023013626322.
Opportunities like this come across only once every few years.This ain’t financial advice…But if you got the cash.Never bet against America
Number of synapses in the human brain by  Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
Some of the most notable ones:
- 1942: Chicago Pile-1: the first human-made nuclear chain reaction.
- 1943: X-10 Graphite Reactor: an intermediate step between the nuclear chain reaction prototype Chicago Pile-1 and the full blown mass production at Hanford site. Located in the Oak Ridge National Laboratory.
- 1944: B Reactor at the Hanford site produced the plutonium used for Trinity and Fat Man
Good documentary about it: Nick Leeson and the Fall of the House of Barings by Adam Curtis (1996).
One is reminded of Annie Dookhan.
This is natural question because both integer factorization and discrete logarithm are the basis for the most popular public-key cryptography systems as of 2020 (RSA and Diffie-Hellman key exchange respectively), and both are NP-intermediate. Why not use something more provenly hard?
- cs.stackexchange.com/questions/356/why-hasnt-there-been-an-encryption-algorithm-that-is-based-on-the-known-np-hard "Why hasn't there been an encryption algorithm that is based on the known NP-Hard problems?"
Ultimate explanation: math.stackexchange.com/questions/776039/intuition-behind-normal-subgroups/3732426#3732426
Only normal subgroups can be used to form quotient groups: their key definition is that they plus their cosets form a group.
One key intuition is that "a normal subgroup is the kernel" of a group homomorphism, and the normal subgroup plus cosets are isomorphic to the image of the isomorphism, which is what the fundamental theorem on homomorphisms says.
Therefore "there aren't that many group homomorphism", and a normal subgroup it is a concrete and natural way to uniquely represent that homomorphism.
The best way to think about the, is to always think first: what is the homomorphism? And then work out everything else from there.
Explicit scalar form of the Maxwell's equations by  Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
For numerical algorithms and to get a more low level understanding of the equations, we can expand all terms to the simpler and more explicit form:
Navier-Stokes existence and smoothness by  Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
Non-primitive total recursive function by  Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
No-Nonsense Quantum Field Theory by Jakob Schwichtenberg (2020) by  Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
This book really tries to recall basic things to ensure that the reader will be able to understand the more advanced ones.
But Ciro Santilli really prefers it when authors error on the side of obvious.
E. Coli Whole Cell Model by Covert Lab Install and first run by  Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
Ciro Santilli 35 Updated 2025-04-24 +Created 1970-01-01
At 7e4cc9e57de76752df0f4e32eca95fb653ea64e4 you basically need to use the Docker image on Ubuntu 21.04 due to pip breaking changes... (not their fault). Perhaps pyenv would solve things, but who has the patience for that?!?!
The Docker setup from README does just work. The image download is a bit tedius, as it requires you to create a GitHub API key as described in the README, but there must be reasons for that.
Once the image is downloaded, you really want to run is from the root of the source tree:This mounts the host source under The meaning of each of the analysis commands is described at Section "Output overview".
sudo docker run --name=wcm -it -v "$(pwd):/wcEcoli" docker.pkg.github.com/covertlab/wholecellecolirelease/wcm-full/wcEcoli, so you can easily edit and view output images from your host. Once inside Docker we can compile, run the simulation, and analyze results with:make clean compile &&
python runscripts/manual/runFitter.py &&
python runscripts/manual/runSim.py &&
python runscripts/manual/analysisVariant.py &&
python runscripts/manual/analysisCohort.py &&
python runscripts/manual/analysisMultigen.py &&
python runscripts/manual/analysisSingle.pyAs a Docker refresher, after you stop the container, e.g. by restarting your computer or running
sudo docker stop wcm, you can get back into it with:sudo docker start wcm
sudo docker run -it wcm bashrunscripts/manual/runFitter.py takes about 15 minutes, and it generates files such as reconstruction/ecoli/dataclasses/process/two_component_system.py (related) which is required to run the simulation, it is basically a part of the build.runSim.py does the main simulation, progress output contains lines of type:Time (s) Dry mass Dry mass Protein RNA Small mol Expected
(fg) fold change fold change fold change fold change fold change
======== ======== =========== =========== =========== =========== ===========
0.00 403.09 1.000 1.000 1.000 1.000 1.000
0.20 403.18 1.000 1.000 1.000 1.000 1.000 2569.18 783.09 1.943 1.910 2.005 1.950 1.963
Simulation finished:
- Length: 0:42:49
- Runtime: 0:09:13 Pinned article: ourbigbook/introduction-to-the-ourbigbook-project
Welcome to the OurBigBook Project! Our goal is to create the perfect publishing platform for STEM subjects, and get university-level students to write the best free STEM tutorials ever.
Everyone is welcome to create an account and play with the site: ourbigbook.com/go/register. We belive that students themselves can write amazing tutorials, but teachers are welcome too. You can write about anything you want, it doesn't have to be STEM or even educational. Silly test content is very welcome and you won't be penalized in any way. Just keep it legal!
Intro to OurBigBook
. Source. We have two killer features:
- topics: topics group articles by different users with the same title, e.g. here is the topic for the "Fundamental Theorem of Calculus" ourbigbook.com/go/topic/fundamental-theorem-of-calculusArticles of different users are sorted by upvote within each article page. This feature is a bit like:
- a Wikipedia where each user can have their own version of each article
- a Q&A website like Stack Overflow, where multiple people can give their views on a given topic, and the best ones are sorted by upvote. Except you don't need to wait for someone to ask first, and any topic goes, no matter how narrow or broad
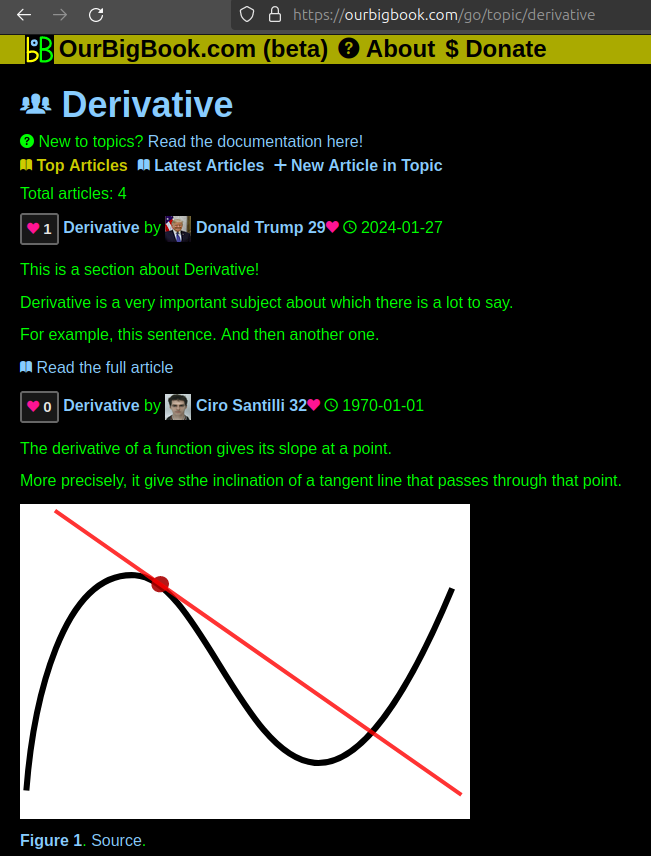
This feature makes it possible for readers to find better explanations of any topic created by other writers. And it allows writers to create an explanation in a place that readers might actually find it.Figure 1. Screenshot of the "Derivative" topic page. View it live at: ourbigbook.com/go/topic/derivativeVideo 2. OurBigBook Web topics demo. Source. - local editing: you can store all your personal knowledge base content locally in a plaintext markup format that can be edited locally and published either:This way you can be sure that even if OurBigBook.com were to go down one day (which we have no plans to do as it is quite cheap to host!), your content will still be perfectly readable as a static site.
- to OurBigBook.com to get awesome multi-user features like topics and likes
- as HTML files to a static website, which you can host yourself for free on many external providers like GitHub Pages, and remain in full control
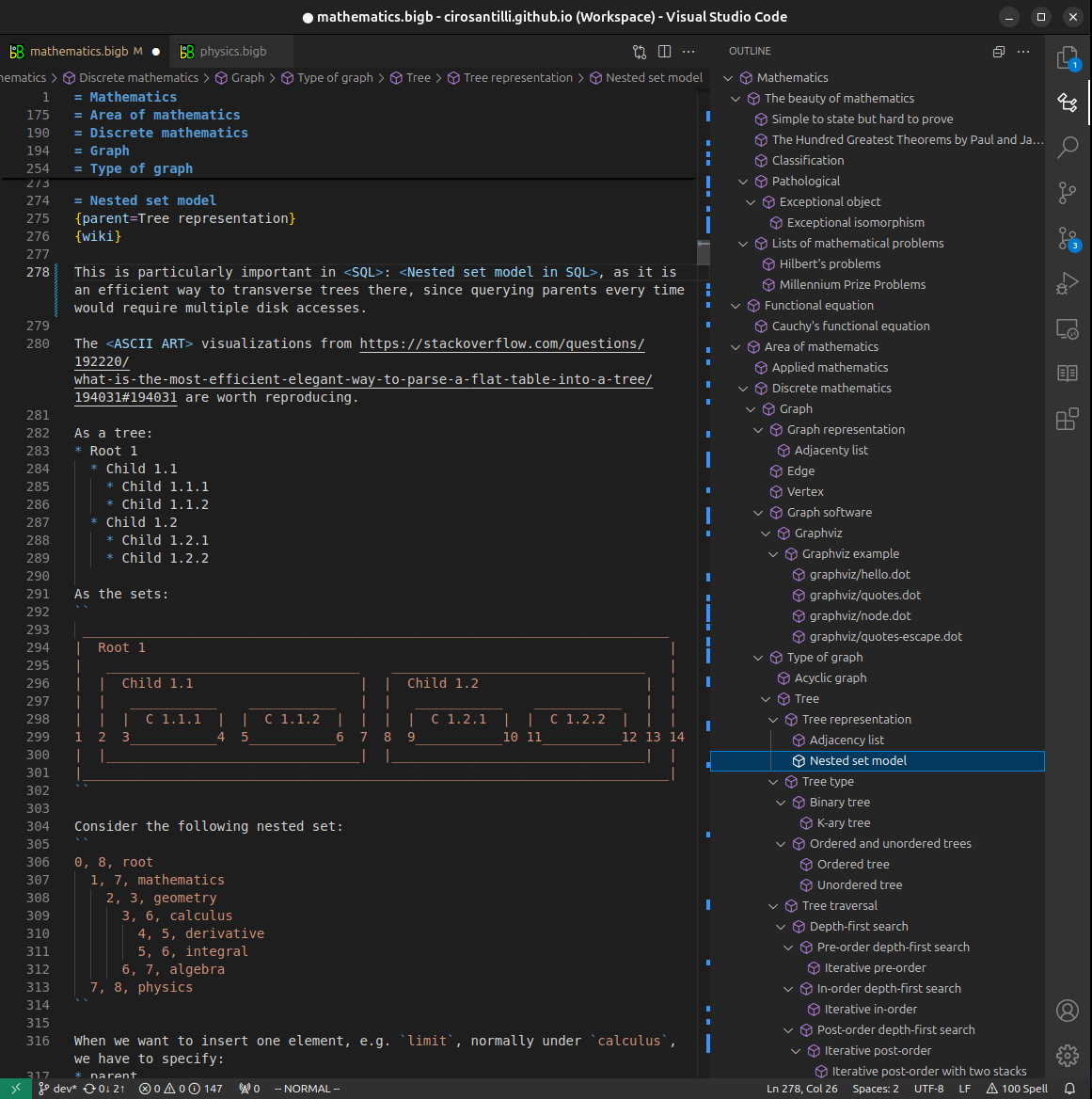
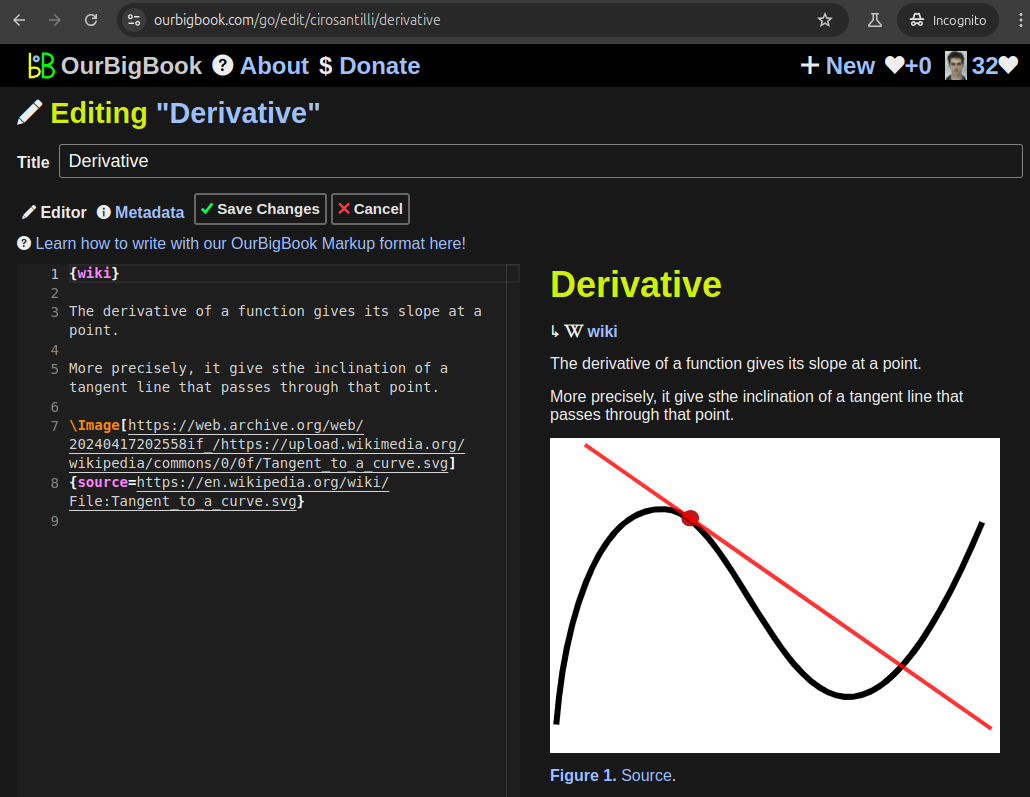
Figure 2. You can publish local OurBigBook lightweight markup files to either OurBigBook.com or as a static website.Figure 3. Visual Studio Code extension installation.Figure 5. . You can also edit articles on the Web editor without installing anything locally. Video 3. Edit locally and publish demo. Source. This shows editing OurBigBook Markup and publishing it using the Visual Studio Code extension. - Infinitely deep tables of contents:
All our software is open source and hosted at: github.com/ourbigbook/ourbigbook
Further documentation can be found at: docs.ourbigbook.com
Feel free to reach our to us for any help or suggestions: docs.ourbigbook.com/#contact